Page 173 - DMTH202_BASIC_MATHEMATICS_II

Basic Mathematics-II

Notes

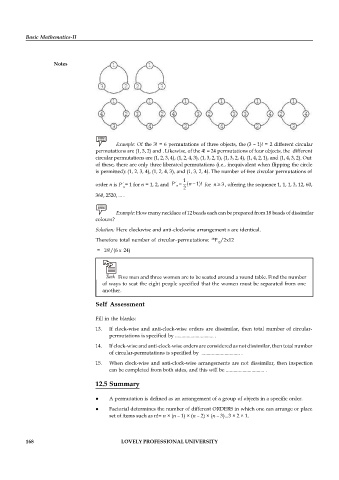
Example: Of the 3! = 6 permutations of three objects, the (3 – 1)! = 2 different circular
permutations are (1, 3, 2) and . Likewise, of the 4! = 24 permutations of four objects, the different
circular permutations are (1, 2, 3, 4), (1, 2, 4, 3), (1, 3, 2, 1), (1, 3, 2, 4), (1, 4, 2, 1), and (1, 4, 3, 2). Out
of these, there are only three liberated permutations (i.e., inequivalent when flipping the circle
is permitted): (1, 2, 3, 4), (1, 2, 4, 3), and (1, 3, 2, 4). The number of free circular permutations of
1
order n is P´ = 1 for n = 1, 2, and ´P  n   1 ! for n  , offering the sequence 1, 1, 1, 3, 12, 60,
3
n
n 2
360, 2520, ... .
Example: How many necklace of 12 beads each can be prepared from 18 beads of dissimilar
colours?
Solution: Here clockwise and anti-clockwise arrangement s are identical.
18
Therefore total number of circular–permutations: P /2x12
12
= 18!/(6 x 24)

Task Five men and three women are to be seated around a round table. Find the number
of ways to seat the eight people specified that the women must be separated from one
another.

Self Assessment

Fill in the blanks:

13. If clock-wise and anti-clock-wise orders are dissimilar, then total number of circular-
permutations is specified by .............................. .
14. If clock-wise and anti-clock-wise orders are considered as not dissimilar, then total number
of circular-permutations is specified by .............................. .
15. When clock-wise and anti-clock-wise arrangements are not dissimilar, then inspection
can be completed from both sides, and this will be .............................. .

12.5 Summary

 A permutation is defined as an arrangement of a group of objects in a specific order.
 Factorial determines the number of different ORDERS in which one can arrange or place
set of items such as n!= n × (n – 1) × (n – 2) × (n – 3)...3 × 2 × 1.

168 LOVELY PROFESSIONAL UNIVERSITY