Page 172 - DMTH202_BASIC_MATHEMATICS_II

Unit 12: Permutation

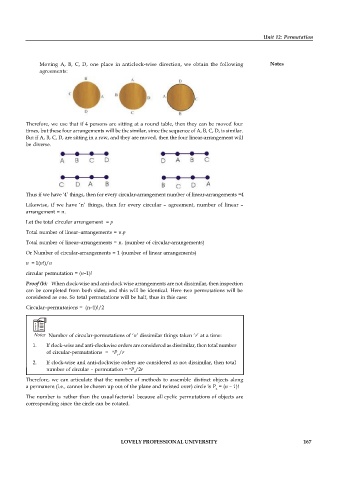
Moving A, B, C, D, one place in anticlock-wise direction, we obtain the following Notes
agreements:

Therefore, we use that if 4 persons are sitting at a round table, then they can be moved four
times, but these four arrangements will be the similar, since the sequence of A, B, C, D, is similar.
But if A, B, C, D, are sitting in a row, and they are moved, then the four linear-arrangement will
be diverse.

Thus if we have ‘4’ things, then for every circular-arrangement number of linear-arrangements =4
Likewise, if we have ‘n’ things, then for every circular – agreement, number of linear –
arrangement = n.
Let the total circular arrangement = p
Total number of linear–arrangements = n.p

Total number of linear–arrangements = n. (number of circular-arrangements)
Or Number of circular-arrangements = 1 (number of linear arrangements)
n = 1(n!)/n
circular permutation = (n–1)!

Proof (b): When clock-wise and anti-clock wise arrangements are not dissimilar, then inspection
can be completed from both sides, and this will be identical. Here two permutations will be
considered as one. So total permutations will be half, thus in this case:
Circular–permutations = (n–1)!/2

Notes Number of circular-permutations of ‘n’ dissimilar things taken ‘r’ at a time:
1. If clock-wise and anti-clockwise orders are considered as dissimilar, then total number
of circular-permutations = P /r
n
r
2. If clock-wise and anti-clockwise orders are considered as not dissimilar, then total
number of circular – permutation = P /2r
n
r
Therefore, we can articulate that the number of methods to assemble distinct objects along
a permanent (i.e., cannot be chosen up out of the plane and twisted over) circle is P = (n – 1)!
n
The number is rather than the usual factorial because all cyclic permutations of objects are
corresponding since the circle can be rotated.

LOVELY PROFESSIONAL UNIVERSITY 167