Page 77 - DMTH202_BASIC_MATHEMATICS_II

Basic Mathematics-II

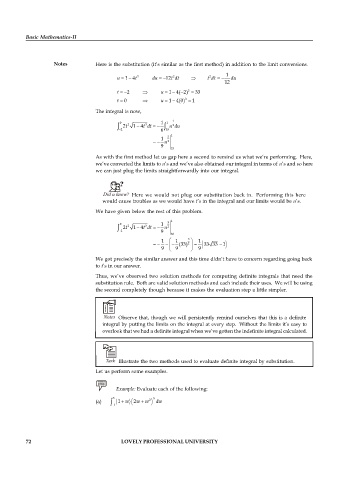
Notes Here is the substitution (it’s similar as the first method) in addition to the limit conversions.
1
2
2

u  1 4t 3 du   12t dt  t dt   du
12
3

t   2  u  1 4( 2)  33

3
t  0  u  1 4(0)  1

The integral is now,
0 2 3 1 1 1 2
2  2t 1 4t dt   33  u du

 6
1
3
1
  u 2
9
33
As with the first method let us gap here a second to remind us what we’re performing. Here,
we’ve converted the limits to u’s and we’ve also obtained our integral in terms of u’s and so here
we can just plug the limits straightforwardly into our integral.
Did u know? Here we would not plug our substitution back in. Performing this here
would cause troubles as we would have t’s in the integral and our limits would be u’s.
We have given below the rest of this problem.
1
0 2 3 1 3 2

2  2t 1 4t dt   u
 9
33
1  1 3  1
2
    (33)    33 33   1

9  9  9
We got precisely the similar answer and this time didn’t have to concern regarding going back
to t’s in our answer.
Thus, we’ve observed two solution methods for computing definite integrals that need the
substitution rule. Both are valid solution methods and each include their uses. We will be using
the second completely though because it makes the evaluation step a little simpler.

Notes Observe that, though we will persistently remind ourselves that this is a definite
integral by putting the limits on the integral at every step. Without the limits it’s easy to
overlook that we had a definite integral when we’ve gotten the indefinite integral calculated.

Task Illustrate the two methods used to evaluate definite integral by substitution.
Let us perform some examples.

Example: Evaluate each of the following:
5 5
(a) 1  1 w 2w w 2  dw


72 LOVELY PROFESSIONAL UNIVERSITY