Page 15 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes Note that it looks just like the graph of sin except it’s translated to the left by /2. That’s because
of the identity cos = sin ( /2 + ). Although we haven’t come across this identity before, it easily
follows from ones that we have seen: cos = cos – = sin ( /2 – (– )) = sin ( /2 + ).

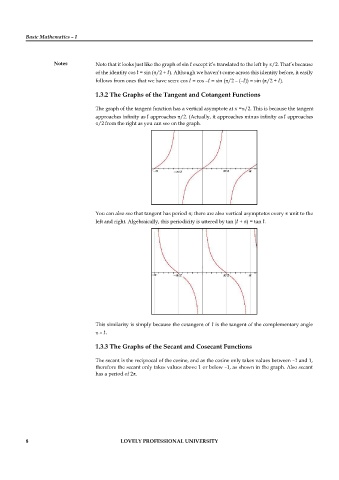
1.3.2 The Graphs of the Tangent and Cotangent Functions

The graph of the tangent function has a vertical asymptote at x = /2. This is because the tangent

approaches infinity as approaches /2. (Actually, it approaches minus infinity as approaches

/2 from the right as you can see on the graph.

You can also see that tangent has period ; there are also vertical asymptotes every unit to the
left and right. Algebraically, this periodicity is uttered by tan ( + ) = tan .

This similarity is simply because the cotangent of is the tangent of the complementary angle
–

1.3.3 The Graphs of the Secant and Cosecant Functions

The secant is the reciprocal of the cosine, and as the cosine only takes values between –1 and 1,
therefore the secant only takes values above 1 or below –1, as shown in the graph. Also secant
has a period of 2 .

8 LOVELY PROFESSIONAL UNIVERSITY