Page 19 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes To solve this, we choose a trigonometric ratio, which involves both AE and DE. Let us choose the
tangent of the angle of elevation.

Now, tan 45° =

i.e., 1 =

Therefore, AE = 10
So the height of the satellite (AB) = (28.5 + 1.5) m = 30 m.

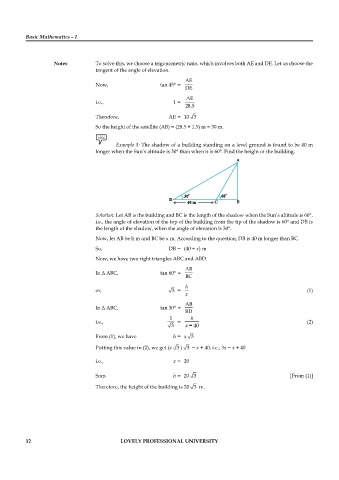
Example 3: The shadow of a building standing on a level ground is found to be 40 m
longer when the Sun’s altitude is 30° than when it is 60°. Find the height of the building.

Solution: Let AB is the building and BC is the length of the shadow when the Sun’s altitude is 60°,
i.e., the angle of elevation of the top of the building from the tip of the shadow is 60° and DB is
the length of the shadow, when the angle of elevation is 30°.
Now, let AB be h m and BC be x m. According to the question, DB is 40 m longer than BC.
So, DB = (40 + x) m
Now, we have two right triangles ABC and ABD.

In Δ ABC, tan 60° =

or, = (1)

In Δ ABC, tan 30° =

i.e., = (2)

From (1), we have h = x

Putting this value in (2), we get (x ) = x + 40, i.e., 3x = x + 40

i.e., x = 20

Som h = 20 [From (1)]
Therefore, the height of the building is 20 m.

12 LOVELY PROFESSIONAL UNIVERSITY