Page 318 - DMTH201_Basic Mathematics-1

Unit 12: Successive Differentiation

Notes

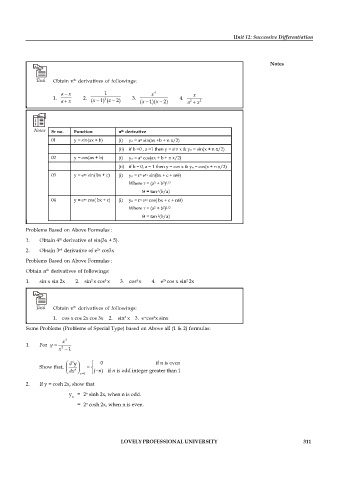
Task Obtain n derivatives of followings:
th
a x 1 x 4 x
1. 2. 2 3. 4.
a x (x 1) (x 2) (x 1)(x 2) a 2 x 2

Notes Sr no. Function n derivative
th
01 y = sin(ax + b) (i) yn = a sin(ax +b + n /2)
n
(ii) if b =0 , a =1 then y = sin x & yn = sin(x + n /2)
02 y = cos(ax + b) (i) yn = a cos(ax + b + n /2)
n
(ii) if b = 0, a = 1 then y = cos x & yn = cos(x + n /2)
ax
ax
n
03 y = e sin( bx + c) (i) yn = r e sin(bx + c + n )
Where r = (a + b )
2
2 1/2
-1
= tan (b/a)
04 y = e cos( bx + c) (i) yn = r e cos( bx + c + n )
ax
n
ax
2
Where r = (a + b )
2 1/2
-1
= tan (b/a)
Problems Based on Above Formulas :
th
1. Obtain 4 derivative of sin(3x + 5).
2x
rd
2. Obtain 3 derivative of e cos3x
Problems Based on Above Formulas :
th
Obtain n derivatives of followings:
4
2x
2
3
2
1. sin x sin 2x 2. sin x cos x 3. cos x 4. e cos x sin 2x
Task Obtain n derivatives of followings:
th
2
-x
1. cos x cos 2x cos 3x 2. sin x 3. e cos x sinx
4
Some Problems (Problems of Special Type) based on Above all (1 & 2) formulas:
x 3
1. For y
x 2 1
n
d y 0 if n is even
Show that,
n
dx n ( n ) if is odd integer greater than 1
x 0
2. If y = cosh 2x, show that
y = 2 sinh 2x, when n is odd.
n
n
n
= 2 cosh 2x, when n is even.

LOVELY PROFESSIONAL UNIVERSITY 311