Page 320 - DMTH201_Basic Mathematics-1
P. 320
Unit 12: Successive Differentiation
Notes
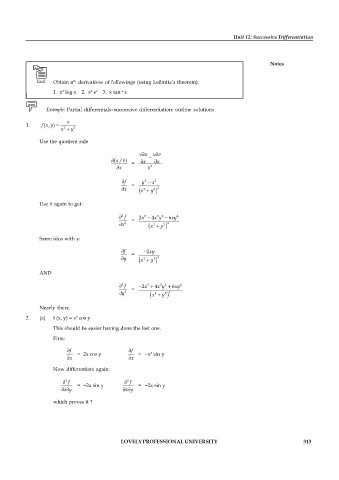
Task Obtain n derivatives of followings (using Leibnitz’s theorem):
th
1. x log x 2. x e x 3. x tan x
2
2
-1
Example: Partial differentials–successive differentiation: outline solutions
x
y
1. f ( , )
x
x 2 y 2
Use the quotient rule
v u u v
( / ) x x
v
u
=
x v 2
f y 2 x 2
=
x x 2 y 2 2
Use it again to get:
2
3
f 2x 5 4x y 2 6xy 4
=
x 2 x 2 y 2 4
Same idea with y:
f 2xy
=
y x 2 y 2 2
AND
2 5 3 2 4
f 2x 4x y 6xy
=
dy 2 x 2 y 2 4
Nearly there.
2
2. (a) f (x, y) = x cos y
This should be easier having done the last one.
First:
f f
2
= 2x cos y = x sin y
x x
Now differentiate again:
2 2
f f
= 2x sin y = 2x sin y
x y x y
which proves it ?
LOVELY PROFESSIONAL UNIVERSITY 313