Page 30 - DMTH202_BASIC_MATHEMATICS_II

Unit 2: Integration by Partial Fraction

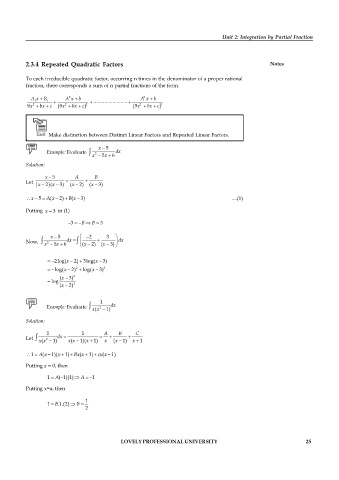
2.3.4 Repeated Quadratic Factors Notes

To each irreducible quadratic factor, occurring n times in the denominator of a proper rational
fraction, there corresponds a sum of n partial fractions of the form.

2
2
A x B 1  A x b            A x b



1
2
2
2

9x  bx c (9x  bx  ) c 2 (9x  bx  ) c 2
Task Make distinction between Distinct Linear Factors and Repeated Linear Factors.
x  5
Example: Evaluate  2 dx
x  5x  6
Solution:
x  5 A B
Let  
x   2 (x  3) (x  2) (x  3)

 x  5  A (x  2) B (x  3) ....(1)
Putting x  in (1)
3
B
 3    B  3

x  5   2 3 
Now,  2 dx      dx
x  5x  6  (x  2) (x  3) 


  2log(x  2) 3log(x  3)
2
  log(x  2)  log(x  3) 3
(x  3) 3
 log
(x  2) 2

1
Example: Evaluate  2 dx
( x x  1)
Solution:

1 1 A B C
Let  2 dx    
( x x  1) ( x x  1)(x  1) x (x  1) x  1


 1  A (x  1)(x  1) Bx (x  1) cx (x  1)
Putting x = 0, then

1  A ( 1)(1)  A   1
Putting x=a, then
1
1  B .1.(2)  B 
2

LOVELY PROFESSIONAL UNIVERSITY 25