Page 111 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

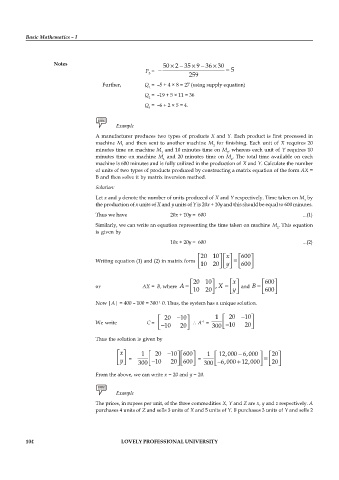
Notes 50 2 35 9 36 30
P = 5
3
259
Further, Q = –5 + 4 × 8 = 27 (using supply equation)
1
Q = –19 + 5 × 11 = 36
2
Q = –6 + 2 × 5 = 4.
3

Example
A manufacturer produces two types of products X and Y. Each product is first processed in
machine M and then sent to another machine M for finishing. Each unit of X requires 20
1 2
minutes time on machine M and 10 minutes time on M , whereas each unit of Y requires 10
1 2
minutes time on machine M and 20 minutes time on M . The total time available on each
1 2
machine is 600 minutes and is fully utilized in the production of X and Y. Calculate the number
of units of two types of products produced by constructing a matrix equation of the form AX =
B and then solve it by matrix inversion method.
Solution:
Let x and y denote the number of units produced of X and Y respectively. Time taken on M by
1
the production of x units of X and y units of Y is 20x + 10y and this should be equal to 600 minutes.
Thus we have 20x + 10y = 600 ...(1)
Similarly, we can write an equation representing the time taken on machine M . This equation
2
is given by
10x + 20y = 600 ...(2)

20 10 x 600
Writing equation (1) and (2) in matrix form
10 20 y 600

20 10 x 600
or AX = B, where A , X and B
10 20 y 600

Now |A| = 400 – 100 = 300 ¹ 0. Thus, the system has a unique solution.

20 10 1 20 10
We write C = A =
–1
10 20 300 10 20
Thus the solution is given by

x 1 20 10 600 1 12,000 6,000 20
y = 300 10 20 600 = 300 6,000 12,000 20

From the above, we can write x = 20 and y = 20.

Example
The prices, in rupees per unit, of the three commodities X, Y and Z are x, y and z respectively. A
purchases 4 units of Z and sells 3 units of X and 5 units of Y. B purchases 3 units of Y and sells 2

104 LOVELY PROFESSIONAL UNIVERSITY