Page 113 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

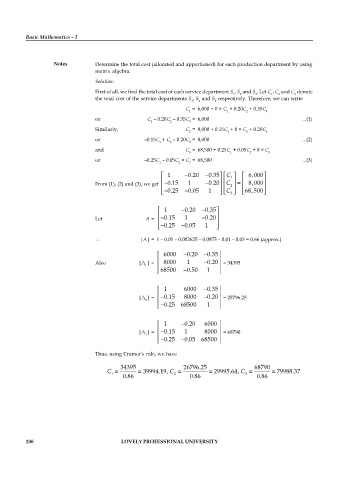
Notes Determine the total cost (allocated and apportioned) for each production department by using
matrix algebra.
Solution:

First of all, we find the total cost of each service department S , S and S . Let C , C and C denote
1 2 3 1 2 3
the total cost of the service departments S , S and S respectively. Therefore, we can write
1 2 3
C = 6,000 + 0 × C + 0.20C + 0.35C
1 1 2 3
or C – 0.20C – 0.35C = 6,000 ...(1)
1 2 3
Similarly, C = 8,000 + 0.15C + 0 × C + 0.20C
2 1 2 3
or –0.15C + C – 0.20C = 8,000 ...(2)
1 2 3
and C = 68,500 + 0.25C + 0.05C + 0 × C
3 1 2 3
or –0.25C – 0.05C + C = 68,500 ...(3)
1 2 3
1 0.20 0.35 C 6,000
1
From (1), (2) and (3), we get 0.15 1 0.20 C 2 8,000
0.25 0.05 1 C 68,500
3

1 0.20 0.35

Let A = 0.15 1 0.20
0.25 0.05 1

|A| = 1 – 0.01 – 0.002625 – 0.0875 – 0.01 – 0.03 = 0.86 (approx.)

6000 0.20 0.35
Also |A | = 8000 1 0.20 = 34395
1
68500 0.50 1

1 6000 0.35
|A | = 0.15 8000 0.20 = 25796.25
2
0.25 68500 1

1 0.20 6000
|A | = 0.15 1 8000 = 68790
3
0.25 0.05 68500

Thus, using Cramer’s rule, we have

34395 26796.25 68790
C 1 39994.19, C 2 29995.64, C 3 79988.37
0.86 0.86 0.86

106 LOVELY PROFESSIONAL UNIVERSITY