Page 46 - DMTH201_Basic Mathematics-1
P. 46
Unit 2: Trigonometric Functions-II
Notes
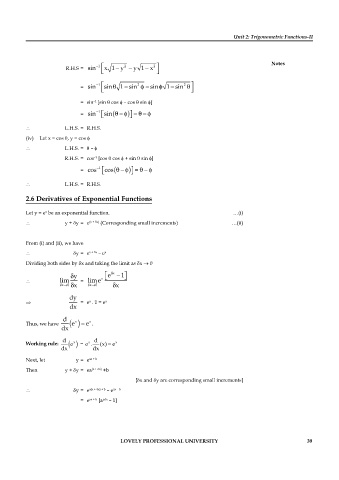
R.H.S =
=
–1
= sin [sin cos – cos sin ]
=
L.H.S. = R.H.S.
(iv) Let x = cos , y = cos
L.H.S. = –
R.H.S. = cos [cos cos + sin sin ]
–1
=
L.H.S. = R.H.S.
2.6 Derivatives of Exponential Functions
x
Let y = e be an exponential function. …(i)
y + y = e (x + x) (Corresponding small increments) …(ii)
From (i) and (ii), we have
y = e x + x – e x
Dividing both sides by x and taking the limit as x 0
=
= e 1 = e x
x
Thus, we have
Working rule: =
Next, let y = e ax + b
Then y + y = ea (x + x) +b
[ x and y are corresponding small increments]
y = e a(x + x) + b – e ax – b
= e ax + b [a – 1]
edx
LOVELY PROFESSIONAL UNIVERSITY 39