Page 49 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes
or =

or, =

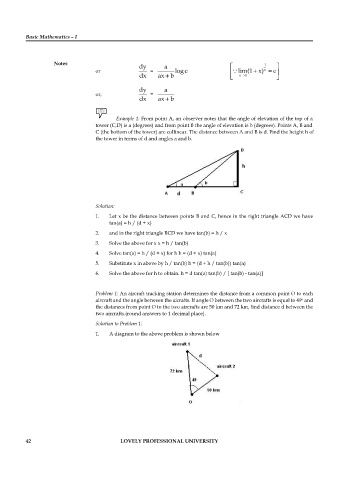
Example 1: From point A, an observer notes that the angle of elevation of the top of a
tower (C,D) is a (degrees) and from point B the angle of elevation is b (degrees). Points A, B and
C (the bottom of the tower) are collinear. The distance between A and B is d. Find the height h of
the tower in terms of d and angles a and b.

Solution:
1. Let x be the distance between points B and C, hence in the right triangle ACD we have
tan(a) = h / (d + x)

2. and in the right triangle BCD we have tan(b) = h / x
3. Solve the above for x x = h / tan(b)
4. Solve tan(a) = h / (d + x) for h h = (d + x) tan(a)
5. Substitute x in above by h / tan(b) h = (d + h / tan(b)) tan(a)

6. Solve the above for h to obtain. h = d tan(a) tan(b) / [ tan(b) - tan(a)]

Problem 1: An aircraft tracking station determines the distance from a common point O to each
o
aircraft and the angle between the aicrafts. If angle O between the two aircrafts is equal to 49 and
the distances from point O to the two aircrafts are 50 km and 72 km, find distance d between the

two aircrafts.(round answers to 1 decimal place).
Solution to Problem 1:

1. A diagram to the above problem is shown below

42 LOVELY PROFESSIONAL UNIVERSITY