Page 47 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes
=

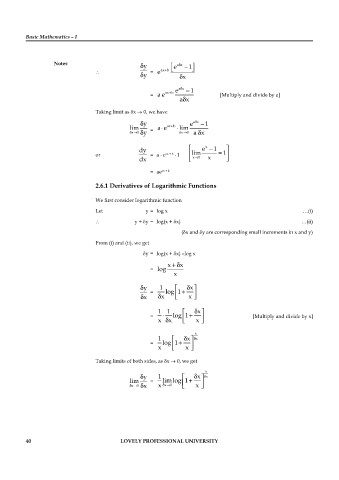
= [Multiply and divide by a]

Taking limit as x 0, we have

=

or = a ∙ e ax + b ∙ 1

= ae ax + b

2.6.1 Derivatives of Logarithmic Functions

We first consider logarithmic function
Let y = log x …(i)
y + y = log(x + x) …(ii)
( x and y are corresponding small increments in x and y)

From (i) and (ii), we get
y = log(x + x) –log x

=

=

= [Multiply and divide by x]

=

Taking limits of both sides, as x 0, we get

=

40 LOVELY PROFESSIONAL UNIVERSITY