Page 230 - DMGT209_QUANTITATIVE_TECHNIQUES_II
P. 230
Unit 11: Multiple Regression and Correlation Analysis
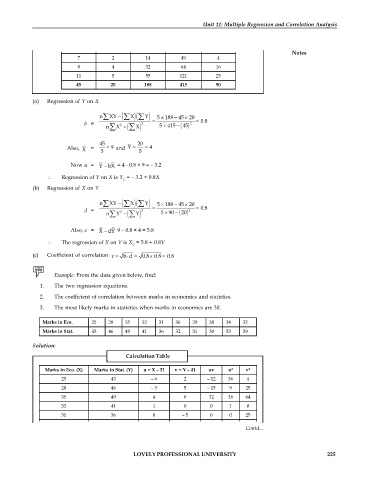
X Y XY X 2 Y 2
10 6 60 100 36
9 3 27 81 9
Notes
7 2 14 49 4
8 4 32 64 16
11 5 55 121 25
45 20 188 415 90
(a) Regression of Y on X
n XY X Y 5 188 45 20
b = 2 2 2 0.8
45
n X X 5 415
45 20
Also, X = 5 9 and Y 5 4
Now a = Y bX = 4 - 0.8 × 9 = – 3.2
Regression of Y on X is Y = – 3.2 + 0.8X
C
(b) Regression of X on Y
n XY X Y 5 188 45 20
d = 2 2 2 0.8
20
n Y Y 5 90
Also, c = X dY 9 – 0.8 × 4 = 5.8
The regression of X on Y is X = 5.8 + 0.8Y
C
(c) Coefficient of correlation r b d 0.8 0.8 0.8
Example: From the data given below, find:
1. The two regression equations.
2. The coefficient of correlation between marks in economics and statistics.
3. The most likely marks in statistics when marks in economics are 30.
Marks in Eco. 25 28 35 32 31 36 29 38 34 32
Marks in Stat. 43 46 49 41 36 32 31 30 33 39
Solution:
Calculation Table
2
Marks in Eco. (X) Marks in Stat. (Y) u = X – 31 v = Y – 41 uv u 2 v
25 43 – 6 2 – 12 36 4
28 46 – 3 5 – 15 9 25
35 49 4 8 32 16 64
32 41 1 0 0 1 0
31 36 0 – 5 0 0 25
36 32 5 – 9 – 45 25 81
Contd...
29 31 – 2 – 10 20 4 100
38 30 7 – 11 – 77 49 121
34 33 3 – 8 – 24 9 64
LOVELY PROFESSIONAL UNIVERSITY 225
32 39 1 – 2 – 2 1 4
Total 10 – 30 – 123 150 488