Page 155 - DMGT404 RESEARCH_METHODOLOGY

Unit 8: Descriptive Statistics

Notes
N
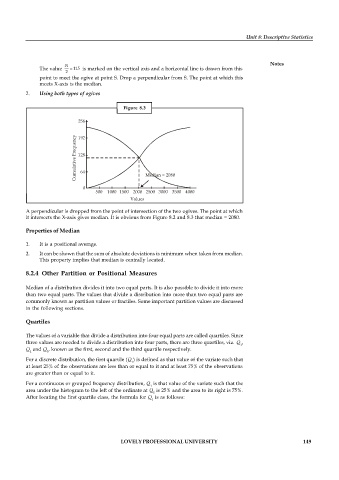
The value  115 is marked on the vertical axis and a horizontal line is drawn from this
2
point to meet the ogive at point S. Drop a perpendicular from S. The point at which this
meets X-axis is the median.
2. Using both types of ogives

Figure 8.3

256

Cumulative Frequency 128
192

64

0 Median = 2080
500 1000 1500 2000 2500 3000 3500 4000
Values

A perpendicular is dropped from the point of intersection of the two ogives. The point at which
it intersects the X-axis gives median. It is obvious from Figure 8.2 and 8.3 that median = 2080.

Properties of Median

1. It is a positional average.
2. It can be shown that the sum of absolute deviations is minimum when taken from median.
This property implies that median is centrally located.

8.2.4 Other Partition or Positional Measures

Median of a distribution divides it into two equal parts. It is also possible to divide it into more
than two equal parts. The values that divide a distribution into more than two equal parts are
commonly known as partition values or fractiles. Some important partition values are discussed
in the following sections.

Quartiles

The values of a variable that divide a distribution into four equal parts are called quartiles. Since
three values are needed to divide a distribution into four parts, there are three quartiles, viz. Q ,
1
Q and Q , known as the first, second and the third quartile respectively.
2 3
For a discrete distribution, the first quartile (Q ) is defined as that value of the variate such that
1
at least 25% of the observations are less than or equal to it and at least 75% of the observations
are greater than or equal to it.
For a continuous or grouped frequency distribution, Q is that value of the variate such that the
1
area under the histogram to the left of the ordinate at Q is 25% and the area to its right is 75%.
1
After locating the first quartile class, the formula for Q is as follows:
1

LOVELY PROFESSIONAL UNIVERSITY 149