Page 160 - DMGT404 RESEARCH_METHODOLOGY

Research Methodology

Notes When Data are in the form of a Grouped Frequency Distribution

The following steps are involved in the computation of mode from a grouped frequency
distribution.
1. Determination of modal class: It is the class in which mode of the distribution lies. If the
distribution is regular, the modal class can be determined by inspection, otherwise, by
method of grouping.

2. Exact location of mode in a modal class (interpolation formula): The exact location of
mode, in a modal class, will depend upon the frequencies of the classes immediately
preceding and following it. If these frequencies are equal, the mode would lie at the
middle of the modal class interval. However, the position of mode would be to the left or
to the right of the middle point depending upon whether the frequency of preceding class
is greater or less than the frequency of the class following it. The exact location of mode
can be done by the use of interpolation formula, developed below:
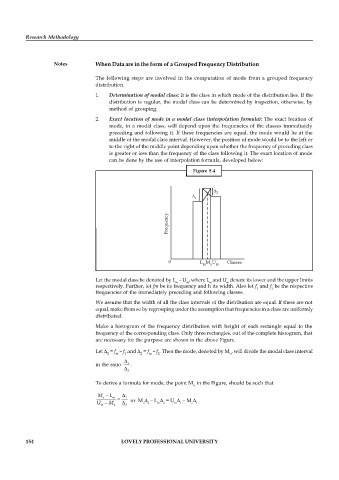
Figure 8.4

 2

1

Frequency

0 L M U Classes
m u m

Let the modal class be denoted by L – U , where L and U denote its lower and the upper limits
m m m m
respectively. Further, let fm be its frequency and h its width. Also let f and f be the respective
1 2
frequencies of the immediately preceding and following classes.
We assume that the width of all the class intervals of the distribution are equal. If these are not
equal, make them so by regrouping under the assumption that frequencies in a class are uniformly
distributed.
Make a histogram of the frequency distribution with height of each rectangle equal to the
frequency of the corresponding class. Only three rectangles, out of the complete histogram, that
are necessary for the purpose are shown in the above Figure.
Let  = f – f and  = f – f . Then the mode, denoted by M , will divide the modal class interval
1 m 1 2 m 2 o

in the ratio 1 .

2
To derive a formula for mode, the point M in the Figure, should be such that
o
M - L 
o m = 1 or M  – L  = U  – M 
U - M  o 2 m 2 m 1 o 1
m o 2

154 LOVELY PROFESSIONAL UNIVERSITY