Page 150 - DMGT404 RESEARCH_METHODOLOGY
P. 150
Research Methodology
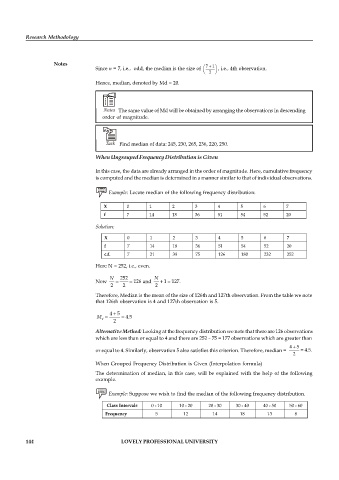
Notes 7 1
Since n = 7, i.e., odd, the median is the size of , i.e., 4th observation.
2
Hence, median, denoted by Md = 20.
Notes The same value of Md will be obtained by arranging the observations in descending
order of magnitude.
Task Find median of data: 245, 230, 265, 236, 220, 250.
When Ungrouped Frequency Distribution is Given
In this case, the data are already arranged in the order of magnitude. Here, cumulative frequency
is computed and the median is determined in a manner similar to that of individual observations.
Example: Locate median of the following frequency distribution:
X 0 1 2 3 4 5 6 7
f 7 14 18 36 51 54 52 20
Solution:
X 0 1 2 3 4 5 6 7
f 7 14 18 36 51 54 52 20
c.f. 7 21 39 75 126 180 232 252
Here N = 252, i.e., even.
N 252 N
Now 126 and 1 127.
2 2 2
Therefore, Median is the mean of the size of 126th and 127th observation. From the table we note
that 126th observation is 4 and 127th observation is 5.
4 5
M 4.5
d
2
Alternative Method: Looking at the frequency distribution we note that there are 126 observations
which are less than or equal to 4 and there are 252 – 75 = 177 observations which are greater than
4 5
or equal to 4. Similarly, observation 5 also satisfies this criterion. Therefore, median = 4.5.
2
When Grouped Frequency Distribution is Given (Interpolation formula)
The determination of median, in this case, will be explained with the help of the following
example.
Example: Suppose we wish to find the median of the following frequency distribution.
Class Intervals 0 - 10 10 - 20 20 - 30 30 - 40 40 - 50 50 - 60
Frequency 5 12 14 18 13 8
144 LOVELY PROFESSIONAL UNIVERSITY