Page 147 - DMGT404 RESEARCH_METHODOLOGY
P. 147
Unit 8: Descriptive Statistics
Notes
– A
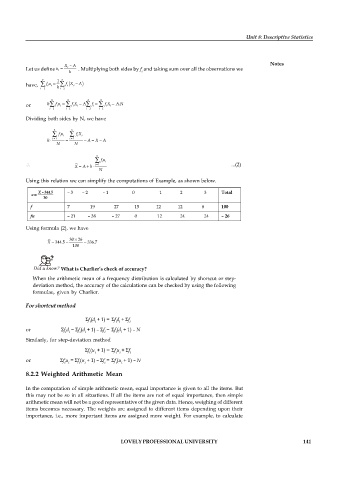
Let us define u i X . Multiplying both sides by f and taking sum over all the observations we
i
h i
n
n
have, i f u f X A
1
i 1 i i h 1 i i
n n n n
h i f u i f X i f i f X . A N
A
or i i i
i 1 i 1 i 1 i 1
Dividing both sides by N, we have
n
n
i f u i f X i
i
h i 1 i 1 A X A
N N
n
i f u i
X A h i 1 ...(2)
N
Using this relation we can simplify the computations of Example, as shown below.
X – 344.5 – 3 – 2 – 1 0 1 2 3 Total
u
30
f 7 19 27 15 12 12 8 100
fu – 21 – 38 – 27 0 12 24 24 – 26
Using formula (2), we have
30 26
X 344.5 336.7
100
Did u know? What is Charlier’s check of accuracy?
When the arithmetic mean of a frequency distribution is calculated by shortcut or step-
deviation method, the accuracy of the calculations can be checked by using the following
formulae, given by Charlier.
For shortcut method
f (d + 1) = f d + f
i i i i i
or f d = f (d + 1) – f = f (d + 1) – N
i i i i i i i
Similarly, for step-deviation method
f (u + 1) = f u + f
i i i i i
or f u = f (u + 1) – f = f (u + 1) – N
i i i i i i i
8.2.2 Weighted Arithmetic Mean
In the computation of simple arithmetic mean, equal importance is given to all the items. But
this may not be so in all situations. If all the items are not of equal importance, then simple
arithmetic mean will not be a good representative of the given data. Hence, weighing of different
items becomes necessary. The weights are assigned to different items depending upon their
importance, i.e., more important items are assigned more weight. For example, to calculate
LOVELY PROFESSIONAL UNIVERSITY 141