Page 257 - DMGT404 RESEARCH_METHODOLOGY

Unit 11: Index Numbers

Notes
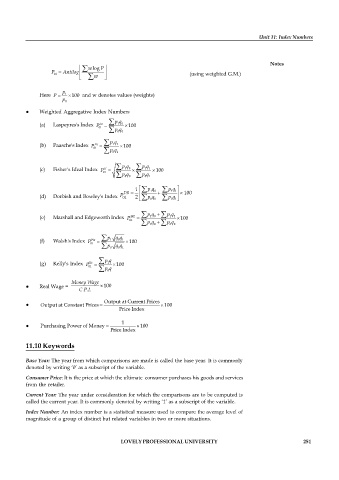
  w log P 
P  Antilog  
01 (using weighted G.M.)
   w  
p
Here P  1  100 and w denotes values (weights)
p
0
 Weighted Aggregative Index Numbers
La  p q
(a) Laspeyres's Index P  1 0  100
01
 p q
0 0
Pa  p q
(b) Paasche's Index P  1 1  100
01
 p q
0 1
1 0 
 p q p q
Fi
(c) Fisher's Ideal Index P   1 1  100
01
 p q p q
0 0 
0 1
1 0 
1   p q p q 
DB    1 1   100
(d) Dorbish and Bowley's Index P 01 2   p q p q
0 0 
0 1  
1 0 
ME  p q  p q
(e) Marshall and Edgeworth Index P  1 1  100
01
 p q  p q
0 0 
0 0
Wa  p q q
(f) Walsh's Index P  1 0 1  100
01
 p 0 q q
0 1
Ke  p q
(g) Kelly's Index P  1  100
01
 p q
0
Money Wage
 Real Wage =  100
I
P
C . . .
Output at Current Prices
 Output at Constant Prices   100
Price Index
1
 Purchasing Power of Money   100
Price Index
11.10 Keywords
Base Year: The year from which comparisons are made is called the base year. It is commonly
denoted by writing ‘0’ as a subscript of the variable.
Consumer Price: It is the price at which the ultimate consumer purchases his goods and services
from the retailer.
Current Year: The year under consideration for which the comparisons are to be computed is
called the current year. It is commonly denoted by writing ‘1’ as a subscript of the variable.
Index Number: An index number is a statistical measure used to compare the average level of
magnitude of a group of distinct but related variables in two or more situations.

LOVELY PROFESSIONAL UNIVERSITY 251