Page 149 - DCOM303_DMGT504_OPERATION_RESEARCH
P. 149
Operations Research
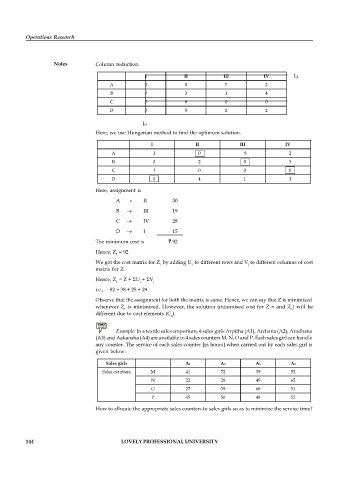
Notes Column reduction.
I IV L 2
II
III
0
A 0 2
7
B 0 3 1 4
C 0 0 0 0
D 0 5 2 2
L 1
Here, we use Hungarian method to find the optimum solution.
I II III IV
A 1 0 8 2
B 0 2 0 3
C 1 0 0 0
D 0 4 1 1
Here, assignment is
A II 30
B III 19
C IV 28
D I 15
The minimum cost is ` 92
Hence, Z = 92
1
We got the cost matrix for Z by adding U to different rows and V to different columns of cost
1 1 j
matrix for Z.
Hence, Z = Z + U + V
1 i j
i.e., 92 = 38 + 25 + 29
Observe that the assignment for both the matrix is same. Hence, we can say that Z is minimized
whenever Z is minimized. However, the solution (minimised cost for Z = and Z ) will be
1 1
different due to cost elements (C ).
ij
Example: In a textile sales emporium, 4 sales girls Arpitha (A1), Archana (A2), Aradhana
(A3) and Aakansha (A4) are available to 4 sales counters M, N, O and P. Each sales girl can handle
any counter. The service of each sales counter [in hours] when carried out by each sales girl is
given below:
Sales girls A1 A2 A3 A4
Sales counters M 41 72 39 52
N 22 29 49 65
O 27 39 60 51
P 45 50 48 52
How to allocate the appropriate sales counters to sales girls so as to minimize the service time?
144 LOVELY PROFESSIONAL UNIVERSITY