Page 24 - DCOM303_DMGT504_OPERATION_RESEARCH

Unit 2: Linear Programming Problems

Notes
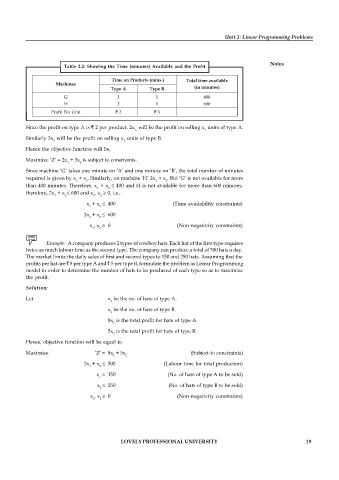
Table 2.2: Showing the Time (minutes) Available and the Profit
Time on Products (mins.) Total time available
Machines
Type A Type B (in minutes)
G 1 1 400
H 2 1 600
Profit Per Unit ` 2 ` 3

Since the profit on type A is ` 2 per product, 2x will be the profit on selling x units of type A.
1- 1
Similarly 3x will be the profit on selling x units of type B.
2 2
Hence the objective function will be,

Maximize ‘Z’ = 2x + 3x is subject to constraints.
1 2
Since machine ‘G’ takes one minute on ‘A’ and one minute on ‘B’, the total number of minutes
required is given by x + x . Similarly, on machine ‘H’ 2x + x . But ‘G’ is not available for more
1 2 1 2
than 400 minutes. Therefore, x + x  400 and H is not available for more than 600 minutes,
1 2
therefore, 2x + x  600 and x , x  0, i.e.,
1 2 1 2,
x + x  400 (Time availability constraints)
1 2
2x + x  600
1 2
x , x  0 (Non-negativity constraints)
1 2

Example: A company produces 2 types of cowboy hats. Each hat of the first type requires
twice as much labour time as the second type. The company can produce a total of 500 hats a day.
The market limits the daily sales of first and second types to 150 and 250 hats. Assuming that the
profits per hat are ` 8 per type A and ` 5 per type B, formulate the problem as Linear Programming
model in order to determine the number of hats to be produced of each type so as to maximize
the profit.

Solution:
Let x be the no. of hats of type A.
1
x be the no. of hats of type B.
2
8x is the total profit for hats of type A.
1
5x is the total profit for hats of type B.
2
Hence, objective function will be equal to

Maximise ‘Z’ = 8x + 5x (Subject to constraints)
1 2
2x + x  500 (Labour time for total production)
1 2
x  150 (No. of hats of type A to be sold)
1
x  250 (No. of hats of type B to be sold)
2
x , x  0 (Non-negativity constraints)
1 2

LOVELY PROFESSIONAL UNIVERSITY 19