Page 26 - DCOM303_DMGT504_OPERATION_RESEARCH
P. 26
Unit 2: Linear Programming Problems
Solution: Notes
Let x be the type of product A
1
x be the type of product B
2
x be the type of product C
3
Therefore the objective function will be,
Maximize ‘Z’ = 3x + 2x + 4x (Subject to constraints)
1 2 3
4x + 3x + 5x 2,000
1 2 3
3x + 2x + 4x 2,500 (Machine hour constraints)
1 2 3
x 150 or 0 x 150
1 1
0 x 200 (Production constraint)
2
0 x 50
3
x , x , x 0 (Non-negativity constraints)
1 2 3
Self Assessment
Multiple Choice Questions:
1. Linear Programming is a
(a) constrained optimization technique
(b) Technique for economic allocation of limited resource
(c) Mathematical technique
(d) All of the above
2. A constraint in a LP model restricts
(a) Value of an objective function (b) Value of a decision variable
(c) Use of the available resources (d) All of the above
3. Constraints of an LP model represents
(a) Limitations
(b) Requirements
(c) Balancing limitations and requirements
(d) All of the above
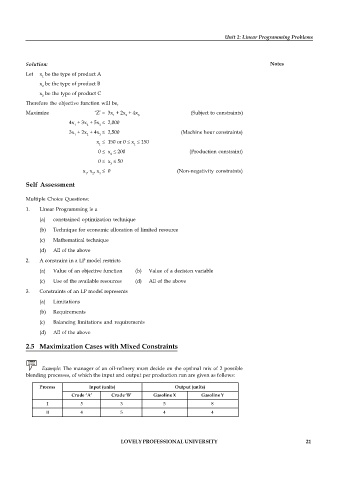
2.5 Maximization Cases with Mixed Constraints
Example: The manager of an oil-refinery must decide on the optimal mix of 2 possible
blending processes, of which the input and output per production run are given as follows:
Process Input (units) Output (units)
Crude ‘A’ Crude ‘B’ Gasoline X Gasoline Y
I 5 3 5 8
II 4 5 4 4
LOVELY PROFESSIONAL UNIVERSITY 21