Page 25 - DCOM303_DMGT504_OPERATION_RESEARCH

Operations Research

Notes
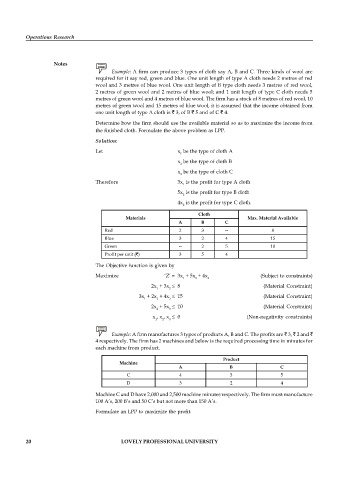
Example: A firm can produce 3 types of cloth say A, B and C. Three kinds of wool are
required for it say red, green and blue. One unit length of type A cloth needs 2 metres of red
wool and 3 metres of blue wool. One unit length of B type cloth needs 3 metres of red wool,
2 metres of green wool and 2 metres of blue wool; and 1 unit length of type C cloth needs 5
metres of green wool and 4 metres of blue wool. The firm has a stock of 8 metres of red wool, 10
metres of green wool and 15 metres of blue wool, it is assumed that the income obtained from
one unit length of type A cloth is ` 3, of B ` 5 and of C ` 4.
Determine how the firm should use the available material so as to maximize the income from
the finished cloth. Formulate the above problem as LPP.
Solution:

Let x be the type of cloth A
1
x be the type of cloth B
2
x be the type of cloth C
3
Therefore 3x is the profit for type A cloth
1
5x is the profit for type B cloth
2
4x is the profit for type C cloth.
2
Cloth
Materials Max. Material Available
A B C
Red 2 3 -- 8
Blue 3 2 4 15
Green -- 2 5 10
Profit per unit (`) 3 5 4
The Objective function is given by

Maximize ‘Z’ = 3x + 5x + 4x (Subject to constraints)
1 2 3
2x + 3x  8 (Material Constraint)
1 2
3x + 2x + 4x  15 (Material Constraint)
1 2 3
2x + 5x  10 (Material Constraint)
2 3
x , x , x  0 (Non-negativity constraints)
1 2 3

Example: A firm manufactures 3 types of products A, B and C. The profits are ` 3, ` 2 and `
4 respectively. The firm has 2 machines and below is the required processing time in minutes for
each machine from product.
Product
Machine
A B C
C 4 3 5
D 3 2 4

Machine C and D have 2,000 and 2,500 machine minutes respectively. The firm must manufacture
100 A’s, 200 B’s and 50 C’s but not more than 150 A’s.
Formulate an LPP to maximize the profit.

20 LOVELY PROFESSIONAL UNIVERSITY