Page 60 - DCOM303_DMGT504_OPERATION_RESEARCH
P. 60
Unit 3: Linear Programming Problem – Simplex Method
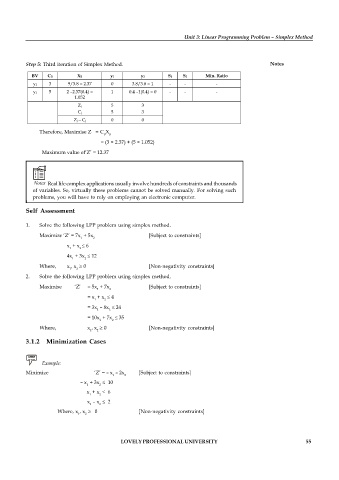
Step 5: Third iteration of Simplex Method. Notes
BV CB XB y1 y2 S1 S2 Min. Ratio
y2 3 9/3.8 = 2.37 0 3.8/3.8 = 1 - - -
y1 5 2 –2.37(0.4) = 1 0.4 –1(0.4) = 0 - - -
1.052
Zj 5 3
Cj 5 3
Zj – Cj 0 0
Therefore, Maximise Z = C X
B B
= (3 × 2.37) + (5 × 1.052)
Maximum value of‘Z’ = 12.37
Notes Real life complex applications usually involve hundreds of constraints and thousands
of variables. So, virtually these problems cannot be solved manually. For solving such
problems, you will have to rely on employing an electronic computer.
Self Assessment
1. Solve the following LPP problem using simplex method.
Maximize ‘Z’ = 7x + 5x [Subject to constraints]
1 2
x + x 6
1 2
4x + 3x 12
1 2
Where, x , x 0 [Non-negativity constraints]
1 2
2. Solve the following LPP problem using simplex method.
Maximise ‘Z’ = 5x + 7x [Subject to constraints]
1 2
= x + x 4
1 2
= 3x – 8x 24
1 2
= 10x + 7x 35
1 2
Where, x , x 0 [Non-negativity constraints]
1 2
3.1.2 Minimization Cases
Example:
Minimize ‘Z’ = – x – 2x [Subject to constraints]
1 2
– x + 3x 10
1 2
x + x 6
1 2
x – x 2
1 2
Where, x , x 0 [Non-negativity constraints]
1 2
LOVELY PROFESSIONAL UNIVERSITY 55