Page 88 - DCOM303_DMGT504_OPERATION_RESEARCH

Unit 3: Linear Programming Problem – Simplex Method

Notes
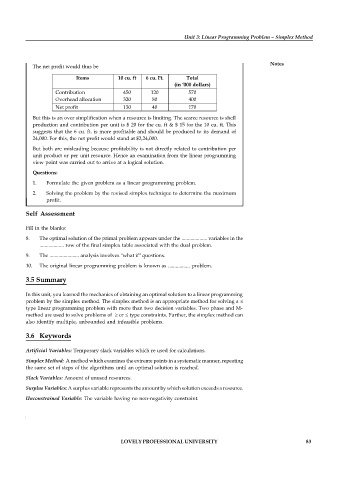
The net profit would thus be
Items 10 cu. ft 6 cu. Ft. Total
(in ‘000 dollars)
Contribution 450 120 570
Overhead allocation 320 80 400
Net profit 130 40 170

But this is an over simplification when a resource is limiting. The scarce resource is shell
production and contribution per unit is $ 20 for the cu. ft & $ 15 for the 10 cu. ft. This
suggests that the 6 cu. ft. is more profitable and should be produced to its demand of
24,000. For this, the net profit would stand at $2,24,000.
But both are misleading because profitability is not directly related to contribution per
unit product or per unit resource. Hence an examination from the linear programming
view point was carried out to arrive at a logical solution.
Questions:

1. Formulate the given problem as a linear programming problem.
2. Solving the problem by the revised simplex technique to determine the maximum
profit.

Self Assessment

Fill in the blanks:
8. The optimal solution of the primal problem appears under the .................... variables in the
................... row of the final simplex table associated with the dual problem.

9. The ....................... analysis involves "what if" questions.
10. The original linear programming problem is known as ................. problem.

3.5 Summary

In this unit, you learned the mechanics of obtaining an optimal solution to a linear programming
problem by the simplex method. The simplex method is an appropriate method for solving a 
type linear programming problem with more than two decision variables. Two phase and M-
method are used to solve problems of  or  type constraints. Further, the simplex method can
also identify multiple, unbounded and infeasible problems.

3.6 Keywords

Artificial Variables: Temporary slack variables which re used for calculations.

Simplex Method: A method which examines the extreme points in a systematic manner, repeating
the same set of steps of the algorithms until an optimal solution is reached.
Slack Variables: Amount of unused resources.

Surplus Variables: A surplus variable represents the amount by which solution exceeds a resource.
Unconstrained Variable: The variable having no non-negativity constraint.

LOVELY PROFESSIONAL UNIVERSITY 83