Page 42 - DMGT515_PERSONAL_FINANCIAL_PLANNING

Unit 2: Time Value of Money

Where, Notes
PVG = PV of growing annuity.
A
CIF = Cash inflows.
g = Growth rate.

I = Discount factor.
n = Duration of the annuity.
Illustration 31
XYZ real estate agency has rented one of their apartment for 5 years at an annual rent of
` 6,00,000 with the stipulation that, rent will increase by 5 per cent every year. If the agency’s
required rate at return is 14 per cent. What is the PV of expected (annuity) rent?

Solution:
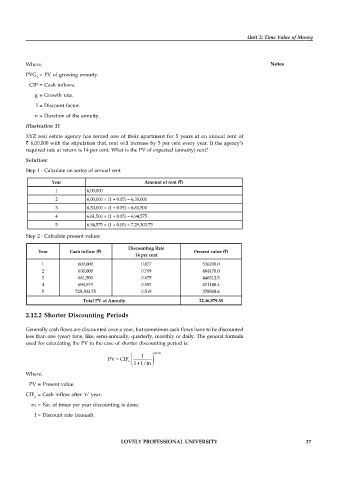
Step 1 : Calculate on series of annual rent

Year Amount of rent (`)
1 6,00,000
2 6,00,000 × (1 + 0.05) = 6,30,000
3 6,30,000 × (1 + 0.05) = 6,61,500
4 6,61,500 × (1 + 0.05) = 6,94,575
5 6,94,575 × (1 + 0.05) = 7,29,303.75
Step 2 : Calculate present values

Discounting Rate
Year Cash inflow (`) Present value (`)
14 per cent
1 600,000 0.877 526200.0
2 630,000 0.769 484470.0
3 661,500 0.675 446512.5
4 694,575 0.592 411188.4
5 729,303.75 0.519 378508.6
Total PV of Annuity 22,46,879.55
2.12.2 Shorter Discounting Periods

Generally cash flows are discounted once a year, but sometimes cash flows have to be discounted
less than one (year) time, like, semi-annually, quarterly, monthly or daily. The general formula
used for calculating the PV in the case of shorter discounting period is:
×
⎛ 1 ⎞ mn
PV = CIF n ⎜ ⎟
1I/m ⎠
⎝ +
Where,
PV = Present value.
CIF = Cash inflow after ‘n’ year.
n
m = No. of times per year discounting is done.
I = Discount rate (annual).

LOVELY PROFESSIONAL UNIVERSITY 37