Page 38 - DMGT515_PERSONAL_FINANCIAL_PLANNING

Unit 2: Time Value of Money

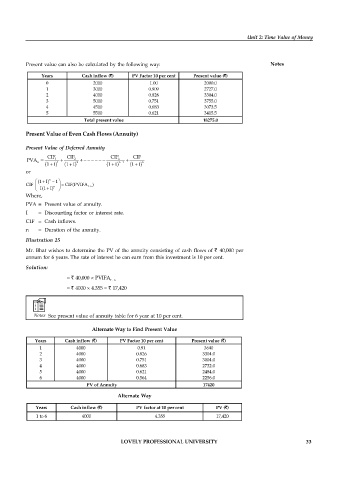
Present value can also be calculated by the following way: Notes
Years Cash inflow (`) PV Factor 10 per cent Present value (`)
0 2000 1.00 2000.0
1 3000 0.909 2727.0
2 4000 0.826 3304.0
3 5000 0.751 3755.0
4 4500 0.683 3073.5
5 5500 0.621 3415.5
Total present value 18275.0

Present Value of Even Cash Flows (Annuity)

Present Value of Deferred Annuity
CIF CIF CIF CIF
PVA = 1 1 + 2 2 +−− −−−− n n 1 + n
n
(1I ) (1 I ) (1I ) − (1 I )
+
+
+
+
or
⎛ (1I+ ) − 1 ⎞
n
CIF ⎜ n ⎟ = CIF(PVIFA 1.n )
+
⎝ ( I1 I ) ⎠
Where,
PVA = Present value of annuity.
I = Discounting factor or interest rate.
CIF = Cash inflows.
n = Duration of the annuity.
Illustration 25
Mr. Bhat wishes to determine the PV of the annuity consisting of cash flows of ` 40,000 per
annum for 6 years. The rate of interest he can earn from this investment is 10 per cent.
Solution:
= ` 40,000 × PVIFA
I . n
= ` 4000 × 4.355 = ` 17,420

Notes See present value of annuity table for 6 year at 10 per cent.

Alternate Way to Find Present Value
Years Cash inflow (`) PV Factor 10 per cent Present value (`)
1 4000 0.91 3640
2 4000 0.826 3304.0
3 4000 0.751 3004.0
4 4000 0.683 2732.0
5 4000 0.621 2484.0
6 4000 0.564 2256.0
PV of Annuity 17420
Alternate Way

Years Cash inflow (`) PV factor at 10 per cent PV (`)
1 to 6 4000 4.355 17,420

LOVELY PROFESSIONAL UNIVERSITY 33