Page 163 - DMGT523_LOGISTICS_AND_SUPPLY_CHAIN_MANAGEMENT

Logistics and Supply Chain Management

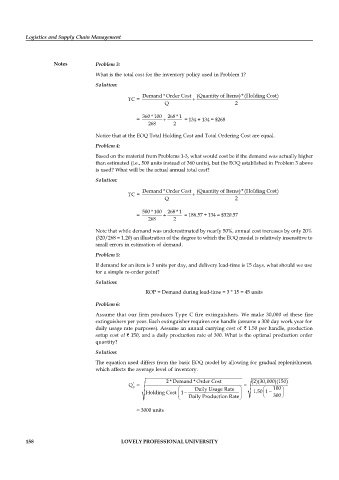
Notes Problem 3:
What is the total cost for the inventory policy used in Problem 1?
Solution:
Demand * Order Cost (Quantity of Items) * (Holding Cost)
TC =
Q 2

360 * 100 268 * 1
= = 134 + 134 = $268
268 2
Notice that at the EOQ Total Holding Cost and Total Ordering Cost are equal.

Problem 4:
Based on the material from Problems 1-3, what would cost be if the demand was actually higher
than estimated (i.e., 500 units instead of 360 units), but the EOQ established in Problem 3 above
is used? What will be the actual annual total cost?
Solution:
Demand * Order Cost (Quantity of Items) * (Holding Cost)
TC =
Q 2

500 * 100 268 * 1
= = 186.57 + 134 = $320.57
268 2
Note that while demand was underestimated by nearly 50%, annual cost increases by only 20%
(320/268 = 1.20) an illustration of the degree to which the EOQ model is relatively insensitive to
small errors in estimation of demand.
Problem 5:

If demand for an item is 3 units per day, and delivery lead-time is 15 days, what should we use
for a simple re-order point?
Solution:

ROP = Demand during lead-time = 3 * 15 = 45 units
Problem 6:
Assume that our firm produces Type C fire extinguishers. We make 30,000 of these fire
extinguishers per year. Each extinguisher requires one handle (assume a 300 day work year for
daily usage rate purposes). Assume an annual carrying cost of ` 1.50 per handle, production
setup cost of ` 150, and a daily production rate of 300. What is the optimal production order
quantity?

Solution:
The equation used differs from the basic EOQ model by allowing for gradual replenishment,
which affects the average level of inventory.

2 * Demand * Order Cost (2)(30,000)(150)
*
Q =
p
Daily Usage Rate 100
Holding Cost 1 1.50 1
Daily Production Rate 300
= 3000 units

158 LOVELY PROFESSIONAL UNIVERSITY