Page 79 - DMGT206_PRODUCTION_AND_OPERATIONS_MANAGEMENT

Production and Operations Management

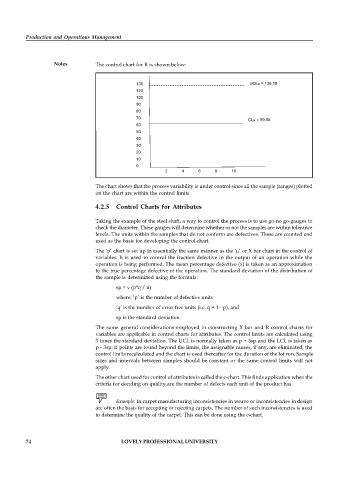
Notes The control chart for R is shown below:

130 UCLR = 126.18
120
100

90
80
70
CLR = 59.56
60
50
40
30
20

10
0
2 4 6 8 10

The chart shows that the process variability is under control since all the sample (ranges) plotted

on the chart are within the control limits.

4.2.5 Control Charts for Attributes

Taking the example of the steel shaft, a way to control the process is to use go-no go-gauges to
check the diameter. These gauges will determine whether or not the samples are within tolerance
levels. The units within the samples that do not conform are defectives. These are counted and
used as the basis for developing the control chart.
The ‘p’ chart is set up in essentially the same manner as the ‘µ’ or X bar chart in the control of
variables. It is used to control the fraction defective in the output of an operation while the
operation is being performed. The mean percentage defective (x) is taken as an approximation
to the true percentage defective of the operation. The standard deviation of the distribution of
the sample is determined using the formula:
sp = v (p*q / n)

where: ‘p’ is the number of defective units
‘q’ is the number of error free units (i.e. q = 1 - p), and
sp is the standard deviation.
The same general considerations employed in constructing X bar and R control charts for
variables are applicable in control charts for attributes. The control limits are calculated using
3 times the standard deviation. The UCL is normally taken as p + 3sp and the LCL is taken as
p - 3sp. If points are found beyond the limits, the assignable causes, if any, are eliminated, the
control limits recalculated and the chart is used thereafter for the duration of the lot run. Sample
sizes and intervals between samples should be constant or the same control limits will not
apply.
The other chart used for control of attributes is called the c-chart. This finds application when the
criteria for deciding on quality are the number of defects each unit of the product has.

Example: In carpet manufacturing inconsistencies in weave or inconsistencies in design
are often the basis for accepting or rejecting carpets. The number of such inconsistencies is used
to determine the quality of the carpet. This can be done using the c-chart.

74 LOVELY PROFESSIONAL UNIVERSITY