Page 123 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 8: Skewness and Kurtosis: Karl Pearson, Bowley, Kelly's Methods

(3) According to Garrett, “A distribution is said to be skewed when the mean and median fall at Notes
different points in the distribution and the balance or centre of gravity is shifted to one side or
the other.
(4) Riggleman and Frisbee have defined skewness as, “Skewness is the lack of symmetry. When a
frequency distribution is plotted on a chart, skewness present in items tends to the disperse
chart more on one side of the mean than on other.”

The measures of ‘Skewness’ tell about the pattern of dispersal of items from an
average, whether it is symmetrical or not. The nature of distribution is further studied
deeply by calculating ‘Moments’ which reveals whether the symmetrical curve is
normal, more flat than normal or more peaked than normal.

From the above discussion it is clear that skewness is the lack of symmetry. Measure of skewness
indicates the difference between the manner in which items are distributed in a particular distribution
compared with symmetrical or normal distribution. In a symmetrical distribution, frequencies go on
increasing upto a point and then begin to decrease in the same fashion. There are various possible
patterns of symmetrical distribution and normal distribution which is bell-shaped is one of these.
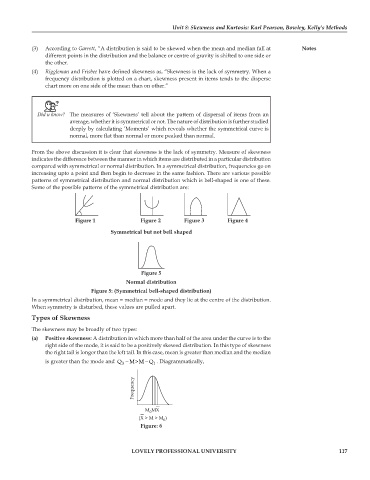
Some of the possible patterns of the symmetrical distribution are:

Figure 1 Figure 2 Figure 3 Figure 4
Symmetrical but not bell shaped

Figure 5
Normal distribution
Figure 5: (Symmetrical bell-shaped distribution)
In a symmetrical distribution, mean = median = mode and they lie at the centre of the distribution.
When symmetry is disturbed, these values are pulled apart.
Types of Skewness
The skewness may be broadly of two types:
(a) Positive skewness: A distribution in which more than half of the area under the curve is to the
right side of the mode, it is said to be a positively skewed distribution. In this type of skewness
the right tail is longer than the left tail. In this case, mean is greater than median and the median
−
is greater than the mode and Q − 3 M>M Q . Diagrammatically,
1
Frequency

M MX
0
(X>M>M )
0
Figure: 6

LOVELY PROFESSIONAL UNIVERSITY 117