Page 125 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 8: Skewness and Kurtosis: Karl Pearson, Bowley, Kelly's Methods

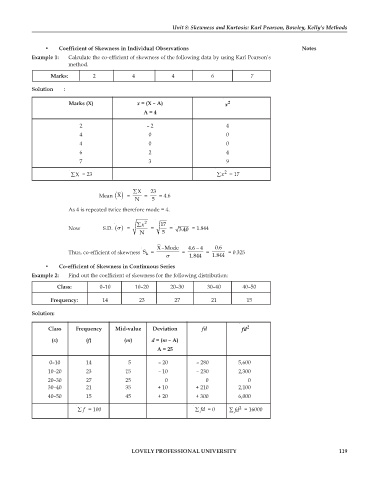
• Coefficient of Skewness in Individual Observations Notes
Example 1: Calculate the co-efficient of skewness of the following data by using Karl Pearson’s
method.
Marks: 2 4 4 6 7

Solution :

Marks (X) x = (X – A) x 2
A = 4

2 – 2 4
4 0 0
4 0 0
6 2 4
7 3 9
2
∑ X = 23 ∑ x = 17

∑ X 23
X
Mean () = = = 4.6
N 5
As 4 is repeated twice therefore mode = 4.

∑ x 2 17
σ
Now S.D. () = = = 3.40 = 1.844
N 5
−
X–Mode 4.6 4 0.6
S
Thus, co-efficient of skewness k = = = = 0.325
σ 1.844 1.844
• Co-efficient of Skewness in Continuous Series
Example 2: Find out the coefficient of skewness for the following distribution:
Class: 0–10 10–20 20–30 30–40 40–50

Frequency: 14 23 27 21 15

Solution:

Class Frequency Mid-value Deviation fd fd 2

(x) (f) (m) d = (m – A)
A = 25

0–10 14 5 – 20 – 280 5,600
10–20 23 15 – 10 – 230 2,300
20–30 27 25 0 0 0
30–40 21 35 + 10 + 210 2,100
40–50 15 45 + 20 + 300 6,000

2
∑ f = 100 ∑ fd = 0 ∑ fd = 16000

LOVELY PROFESSIONAL UNIVERSITY 119