Page 129 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 8: Skewness and Kurtosis: Karl Pearson, Bowley, Kelly's Methods

Notes
×
×
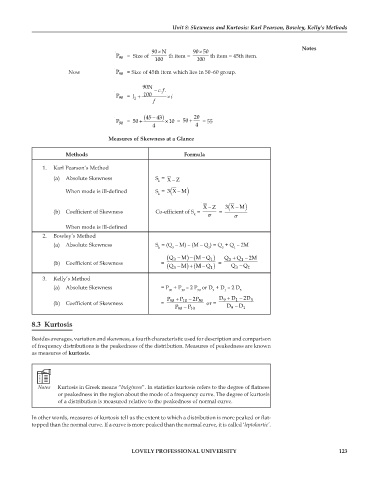
90 N 90 50
P 90 = Size of 100 th item = 100 th item = 45th item.
Now P 90 = Size of 45th item which lies in 50–60 group.

90N − cf
..
P 90 = l + 100 i ×
1
f
( − )45 43 20
P 90 = 50 + × 10 = 50 + = 55
4 4

Measures of Skewness at a Glance

Methods Formula

1. Karl Pearson’s Method
(a) Absolute Skewness S = XZ
−
k
When mode is ill-defined S = ( −3X M )
k
−
XZ ( 3X M )
−
(b) Coefficient of Skewness Co-efficient of S = =
k σ σ
When mode is ill-defined
2. Bowley’s Method
(a) Absolute Skewness S = (Q – M) – (M – Q ) = Q + Q – 2M
k 3 1 3 1
(Q − M − ) ( 3 − 1 )M Q 3 + Q 1 − Q 2M
(b) Coefficient of Skewness = ( M + )Q − ( 3 − 1 )M Q = Q 3 − Q 1

3. Kelly’s Method
(a) Absolute Skewness = P + P – 2 P or D + D – 2 D
90 10 50 9 1 5
(b) Coefficient of Skewness = 90 + P 10 − P 2P 50 or = 9 + D 1 − D 2D 5
P 90 − P 10 D 9 − D 1

8.3 Kurtosis

Besides averages, variation and skewness, a fourth characteristic used for description and comparison
of frequency distributions is the peakedness of the distribution. Measures of peakedness are known
as measures of kurtosis.

Kurtosis in Greek means “bulginess”. In statistics kurtosis refers to the degree of flatness
or peakedness in the region about the mode of a frequency curve. The degree of kurtosis
of a distribution is measured relative to the peakedness of normal curve.

In other words, measures of kurtosis tell us the extent to which a distribution is more peaked or flat-
topped than the normal curve. If a curve is more peaked than the normal curve, it is called ‘leptokurtic’.

LOVELY PROFESSIONAL UNIVERSITY 123