Page 130 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Statistical Methods in Economics

Notes In such a case the items are more closely bunched around the mode. On the other hand, if a curve is
more flat-topped than the normal curve, it is called ‘platykurtic’. The normal curve itself is known as
‘mesokurtic’. The condition of peakedness or flat-toppedness itself is known as kurtosis or excess. The
concept of kurtosis is rarely used in elementary statistics.
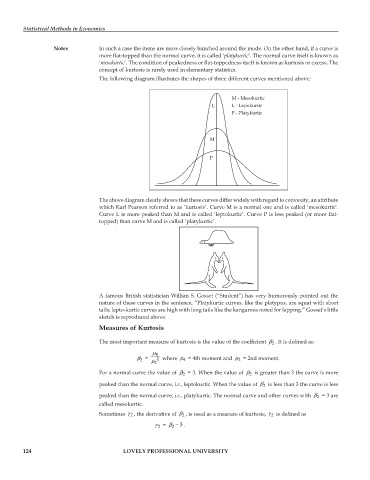
The following diagram illustrates the shapes of three different curves mentioned above:

M - Mesokurtic
L L - Lepokurtic
P - Platykurtic

M

P

The above diagram clearly shows that these curves differ widely with regard to convexity, an attribute
which Karl Pearson referred to as ‘kurtosis’. Curve M is a normal one and is called ‘mesokurtic’.
Curve L is more peaked than M and is called ‘leptokurtic’. Curve P is less peaked (or more flat-
topped) than curve M and is called ‘platykurtic’.

A famous British statistician Willian S. Gosset (“Student”) has very humorously pointed out the
nature of these curves in the sentence, “Platykurtic curves, like the platypus, are squat with short
tails; lepto-kurtic curves are high with long tails like the kangaroos noted for lapping.” Gosset’s little
sketch is reproduced above.
Measures of Kurtosis

The most important measure of kurtosis is the value of the coefficient β . It is defined as:
2
μ 4
β = μ 2 2 where μ = 4th moment and μ = 2nd moment.
4
2
2
For a normal curve the value of β = 3. When the value of β is greater than 3 the curve is more
2
2
peaked than the normal curve, i.e., leptokurtic. When the value of β is less than 3 the curve is less
2
peaked than the normal curve, i.e., platykurtic. The normal curve and other curves with β = 3 are
2
called mesokurtic.
Sometimes γ , the derivative of β , is used as a measure of kurtosis, γ is defined as
2
2
2
γ = β − 3 .
2
2
124 LOVELY PROFESSIONAL UNIVERSITY