Page 75 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_HINDI

bdkbZμ5 % ekè;] ekfè;dk rFkk cgqyd osQ vuqiz;ksx

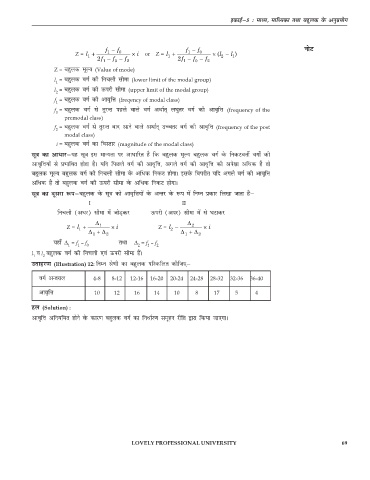
f − f f − f uksV
Z = l + f 2 1 − 1 f − 0 0 f 2 × i or Z = l + f 2 1 1 − f − 0 0 f 2 × l − ( 2 l )
1
1
1
Z = cgqyd ewY; (Value of mode)
l = cgqyd oxZ dh fupyh lhek (lower limit of the modal group)
1
l = cgqyd oxZ dh Åijh lhek (upper limit of the modal group)
2
f = cgqyd oxZ dh vko`fÙk (freqency of modal class)
1
f = cgqyd oxZ ls rqjUr igys okys oxZ vFkkZr~ y?kqrj oxZ dh vko`fÙk (frequency of the
0
premodal class)
f = cgqyd oxZ ls rqjUr ckn vkus okys vFkkZr~ mPprj oxZ dh vko`fÙk (frequency of the post
2
modal class)
i = cgqyd oxZ dk foLrkj (magnitude of the modal class)

lw=k dk vk/kjμ;g lw=k bl ekU;rk ij vk/kfjr gS fd cgqyd ewY; cgqyd oxZ osQ fudVorhZ oxks± dh
vko`fÙk;ksa ls izHkkfor gksrk gSA ;fn fiNys oxZ dh vko`fÙk] vxys oxZ dh vko`fÙk dh vis{kk vf/d gS rks
cgqyd ewY; cgqyd oxZ dh fupyh lhek osQ vf/d fudV gksxkA blosQ foijhr ;fn vxys oxZ dh vko`fÙk
vf/d gS rks cgqyd oxZ dh Åijh lhek osQ vf/d fudV gksxkA

lw=k dk nwljk :iμcgqyd osQ lw=k dks vko`fÙk;ksa osQ vUrj osQ :i esa fuEu izdkj fy[kk tkrk gSμ
I II

fupyh (v/j) lhek esa tksM+dj Åijh (vij) lhek esa ls ?kVkdj
Δ Δ
Z = l + 1 × i Z = l − 2 × i
1
2
Δ 1 + Δ 2 Δ 1 + Δ 2
;gk¡ Δ = f – f 0 rFkk Δ = f – f 2
2
1
1
1
l o l cgqyd oxZ dh fupyh ,oa Åijh lhek gSaA
1
2
mnkgj.k (Illustration) 12: fuEu Js.kh dk cgqyd ifjdfyr dhft,μ
oxZ vUrjky 4-8 8-12 12-16 16-20 20-24 24-28 28-32 32-36 36-40
vko`fÙk 10 12 16 14 10 8 17 5 4

gy (Solution) :
vko`fÙk vfu;fer gksus osQ dkj.k cgqyd oxZ dk fu/kZj.k lewgu jhfr }kjk fd;k tk,xkA

LOVELY PROFESSIONAL UNIVERSITY 69