Page 316 - DMTH401_REAL ANALYSIS

Real Analysis

Notes k
Take U =  n 1 I . Note that m(O \ U) = m(O) – m(U) < /2, so
=
n
m(U  E) = m(U\E) + m(E\U)
 m(O\E) +m(O\U)
 
< + = .
2 2
The finiteness assumption is essential here. The above result is false if we allow E to have
infinite measure. A counter example is E =  ¥ n 1 (2n, 2n + 1).
=
To show (6)  (2) (without finiteness assumption m*(E) < ¥)
Let  > 0 be given and U be a finite union of open intervals. Then m*(E\U) <, we take an open
set O  E\U such that m*(O) <  (how to do this?). Then O = U  O is an open set containing
E with m*(O\E)  m*(U\E) + m*(O) < 2 .

Task Let A  , prove that there is a measurable set B  A with m*(A) = m*(B).

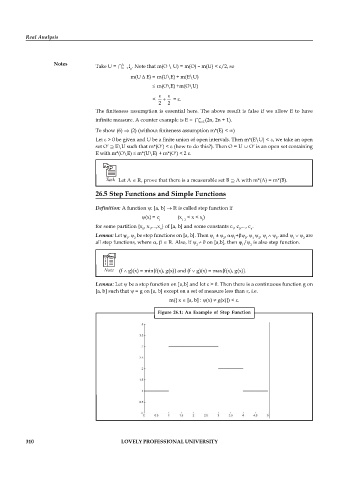
26.5 Step Functions and Simple Functions

Definition: A function : [a, b]   is called step function if
(x) = c (x < x < x )
i i–1 i
for some partition {x , x ,...,x } of [a, b] and some constants c , c ,..., c .
0 1 n 1 2 n
Lemma: Let  ,  be step functions on [a, b]. Then  ±  ,  + ,   ,    , and    are
1 2 1 2 1 2 1 2 1 2 1 2
all step functions, where ,   . Also, if   0 on [a,b], then  / is also step function.
2 1 2

Note (f  g)(x) = min{f(x), g(x)} and (f  g)(x) = max{f(x), g(x)}.

Lemma: Let  be a step function on [a,b] and let  > 0. Then there is a continuous function g on
[a, b] such that  = g on [a, b] except on a set of measure less than , i.e.
m({ x  [a, b] :(x)  g(x)}) < .

Figure 26.1: An Example of Step Function

310 LOVELY PROFESSIONAL UNIVERSITY