Page 128 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Unit 12: Fundamental Theorem of Algebra

Poles of f(z) are z = 1 (order four) and z = 2, 3 (simple) Notes
Therefore,

z 3
Res (z = 2) = lim (z–2) f(z) = lim = –8
z 2 z 2 (z 1) (z 3) 4 

27
Res (z = 3) = lim (z - 3) f(z) =
z 3 16

z 3
For z = 1, we take (z) =
(z 2)(z 3)


where f(z) =  (z) and thus, Res (z = 1) =  3 (1)
(z 1) 4 | 3


8 27
Now, f(z) = z + 5 – 
z 2 z 3


48 162
 (z) = 
3
(z 2) 4 (z 3) 4


303
 (1) =
3
8
Thus,
Res (z = 1) = 303  101
8 3 16
|
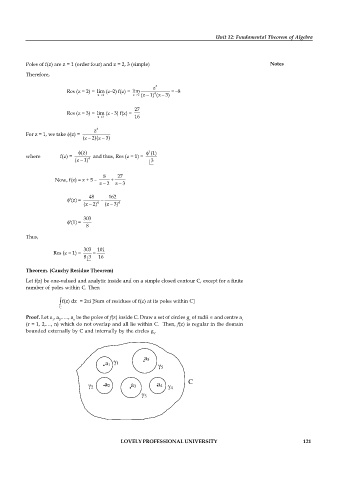
Theorem. (Cauchy Residue Theorem)
Let f(z) be one-valued and analytic inside and on a simple closed contour C, except for a finite
number of poles within C. Then

 f(z) dz = 2i [Sum of residues of f(z) at its poles within C]
C
Proof. Let a , a , ., a be the poles of f(z) inside C. Draw a set of circles g of radii  and centre a r
r
n
1
2
(r = 1, 2, , n) which do not overlap and all lie within C. Then, f(z) is regular in the domain
bounded externally by C and internally by the circles g .
r

a 1  1 a 5
 5

 2 a 2 a 3 a 4  4 C
 3

LOVELY PROFESSIONAL UNIVERSITY 121