Page 145 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

Notes analytic continuation of (f , D ) for K = 1, 2 ., n-1 and z(t)  D for t  t  t , K = 1, 2, , n then
K-1
K
K
K
K
(f , D ) is said to be analytic continuation of (f , D ) along the curve .
n n 1 1
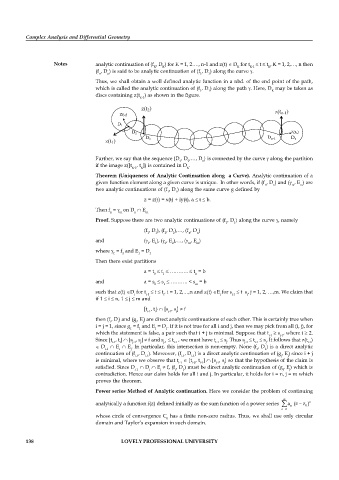
Thus, we shall obtain a well defined analytic function in a nbd. of the end point of the path,
which is called the analytic continuation of (f , D ) along the path . Here, D may be taken as
1
1
K
discs containing z(t ) as shown in the figure.
K-1
Z(t 2)
Z(t n-1)
Z(t 0)

D 1

D 2 Z(t n )
D 3 D n1 D n
Z(t 1)
Further, we say that the sequence {D , D , , D } is connected by the curve  along the partition
2
n
1
if the image z([t , t ]) is contained in D .
K
K-1
K
Theorem (Uniqueness of Analytic Continuation along a Curve). Analytic continuation of a
given function element along a given curve is unique. In other words, if (f , D ) and ( , E ) are
m
n
m
n
two analytic continuations of (f , D ) along the same curve g defined by
1
1
z = z(t) = x(t) + iy(t), a  t  b.
Then f =  on D  E m
m
n
n
Proof. Suppose there are two analytic continuations of (f , D ) along the curve , namely
1
1
(f , D ), (f , D ), , (f , D )
2
2
1
n
n
1
and ( , E ), ( , E ), , ( , E )
1
1
m
m
2
2
where  = f and E = D 1
1
1
1
Then there exist partitions
a = t  t  ..  t = b
0
1
n
and a = s  s  . < s = b
0
1
m
such that z(t) D for t  t  t , i = 1, 2, ,n and z(t) E for s  t s, j = 1, 2, ,m. We claim that
i
i
j
i-1
j
j-1
if 1  i  n, 1  j  m and
[t , t )  [s , s]  f
j-1
i-1
j
i
then (f , D ) and (g , E) are direct analytic continuations of each other. This is certainly true when
j
i
i
i
i = j = 1, since g = f and E = D . If it is not true for all i and j, then we may pick from all (i, j), for
1
1
1
1
which the statement is false, a pair such that i + j is minimal. Suppose that t  s , where i  2.
j-1
i-1
Since [t , t ]  [s , s]  f and s  t , we must have t  s. Thus s  t  s. It follows that z(t )
i-1
j-1
j-1
j
i-1
i
i-1
i-1
j
j-1
i-1
j
 D  E  E. In particular, this intersection is non-empty. None (f , D ) is a direct analytic
i-1
i
1
i
j
continuation of (f , D ). Moreover, (f , D ) is a direct analytic continuation of (g, E) since i + j
j
j
i-1
i-1
i-1
i-1
is minimal, where we observe that t  [t , t ]  [s , s] so that the hypothesis of the claim is
i-1
i-2
i-1
j
j-1
satisfied. Since D  D  E  f, (f , D ) must be direct analytic continuation of (g, E) which is
j
j
j
i
i-1
1
i
contradiction. Hence our claim holds for all i and j. In particular, it holds for i = n, j = m which
proves the theorem.
Power series Method of Analytic continuation. Here we consider the problem of continuing

analytically a function f(z) defined initially as the sum function of a power series  a (z – z ) n
0
n
n 0

whose circle of convergence C has a finite non-zero radius. Thus, we shall use only circular
0
domain and Taylor’s expansion in such domain.
138 LOVELY PROFESSIONAL UNIVERSITY