Page 147 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

Notes But and thus (2) becomes


 (z a) n 1   n
 n 1 =    z a   (4)
n 0 (1 a)  1 a n 0 1 a 

 

z a

Clearly, (3) converges for  1
1 a

and its circle of convergence C is given by |z – a| = |1 – a|. It follows from the inequality (2) that
1
C goes beyond C and hence (4) provides an analytic continuation of f(z) outside C , since we
0
1
0
note that the sum function of (4) is also (1-z) .
-1
On the other hand, if a be a real point inside C such that 0 < a < 1, C touches C at z = 1, which is,
0
1
0
therefore, a singularity of the complete analytic function obtained by analytic continuation
of f(z).
Example: Show that the function
1 z z 2
f(z) =    ...
a a 2 a 3
can be continued analytically.
Solution. We have,
1 z z 2
f(z) =    ... (5)
a a 2 a 3
This series converges within the circle C defined by |z| = |a| and has the sum
0
1/a 1
f(z) = 
1 z/a a z


The only singularity of f(z) on C is at z = a. Hence the analytic continuation of f(z) beyond C is
0
0
possible. For this purpose we take a point z = b not lying on the line segment joining z = 0 and
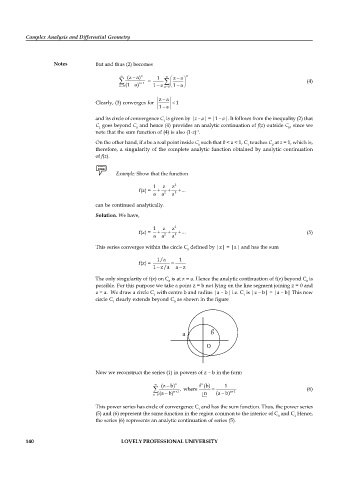
z = a. We draw a circle C with centre b and radius |a – b| i.e. C is |z – b| = |a – b| This new
1
1
circle C clearly extends beyond C as shown in the figure
0
1

a b

O

Now we reconstruct the series (1) in powers of z – b in the form

n
 (z b) n f (b) 1

 n 1 , where  n 1 (6)

n 0 (a b)  | n (a b) 

This power series has circle of convergence C and has the sum function. Thus, the power series
1
(5) and (6) represent the same function in the region common to the interior of C and C Hence,
1
0
the series (6) represents an analytic continuation of series (5).
140 LOVELY PROFESSIONAL UNIVERSITY