Page 151 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

Notes
 1  1 a

Thus, the circle C has the centre  ,0  and radius .
2


 a(2 a)  a(2 a)
Since the two circles depend upon a, where we shall assume that a > 0, we have the following
cases
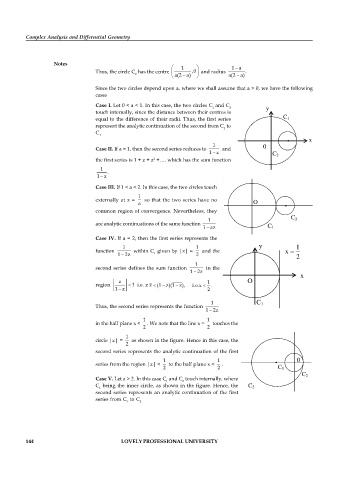
Case I. Let 0 < a < 1. In this case, the two circles C and C 2
1
touch internally, since the distance between their centres is y
equal to the difference of their radii. Thus, the first series C 1
represent the analytic continuation of the second from C to
2
C 1
x
1
Case II. If a = 1, then the second series reduces to and 0
1 z C 2

the first series is 1 + z + z + . which has the sum function
2
1 .
1 z


Case III. If 1 < a < 2. In this case, the two circles touch
1
externally at z = so that the two series have no
a O
common region of convergence. Nevertheless, they
1 C 2
are analytic continuations of the same function
1 az C 1

Case IV. If a = 2, then the first series represents the
1 1 y 1
function within C given by |z| = and the x 
1 2z 1 2 2

1
second series defines the sum function in the
1 2z

x
z 1 O
region  1 i.e. z z  (1 z)(1 z), i.e.x  .


1 z 2

1
Thus, the second series represents the function C 1
1 2z

1 1
in the half plane x < . We note that the line x = touches the
2 2
1
circle |z| = as shown in the figure. Hence in this case, the
2
second series represents the analytic continuation of the first
1 1 0
series from the region |z| < to the half plane x < .
2 2 C 1
C 2
Case V. Let a > 2. In this case C and C touch internally, where
2
1
C being the inner circle, as shown in the figure. Hence, the C 2
1
second series represents an analytic continuation of the first
series from C to C 2
1

144 LOVELY PROFESSIONAL UNIVERSITY