Page 153 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

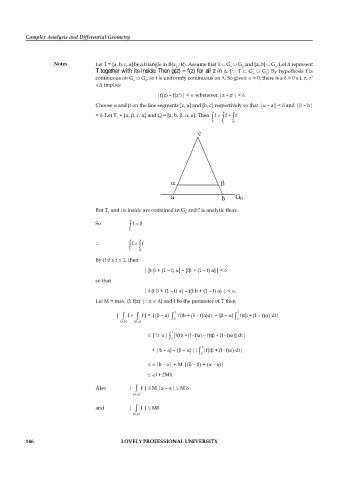
Notes Let T = [a, b, c, a] be a triangle in B(x ; R). Assume that T  G  G and [a, b]  G . Let  represent
+
0
0
0
T together with its inside. Then g(z) = f(z) for all z in . [ T  G  G ] By hypothesis f is
+ 0
continuous on G  G , so f is uniformly continuous on . So given  > 0, there is a  > 0 s.t. z, z
0
+
 implies
|f(z) – f(z)| <  whenever |z – z| < .
Choose  and  on the line segments [c, a] and [b, c] respectively so that | – a| <  and | – b|

< . Let T = [, , c, ] and Q = [a, b, , , a]. Then f   f   f
1
T 1 T Q
c

 
a b G 0

But T and its inside are contained in G and f is analytic there.
+
1
So  f  0
1 T

  f   f
T Q
By if 0  t  1, then
| [t  + (1 – t) ] – [t + (1 – t) a]| < 
so that

| f (t  + (1 – t) ) – f(t b + (1 – t) a) | < .
Let M = max. {1 f(z) | : z  } and l be the perimeter of T then

|  f   f| = |(b – a) 0  1 f(tb + (1 – t)a)dt – (– ) 0  1 f(t + (1 – t) ) dt|


[a,b] [ , ]


 | b -a| 0  1 [f(tb +(1–t)a) – f(t + (1–t) )] dt|


+ |b – a) – ( – )|| 0  1 |f(t + (1–t) ) dt|


 |b – a| + M |(b –) + ( – a)|
 l + 2M.

Also |  f |  M |a – |  M 
[ ,a]

and |  f |  M.
[b, ]


146 LOVELY PROFESSIONAL UNIVERSITY