Page 149 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

Notes 
Example: Show that the power series  z 3n cannot be continued analytically beyond
n 0

the circle |z| = 1
Solution. Here |u (z)| = |z | = |z | = |z| 3
1/n
1/n
3n
3
n
So the series is convergent if |z| < 1
 Circle of convergence is |z| = 1 Now take the point P at z = r e 2 ip /3q , r > 1 and then proceeds

as in the above two examples.
Example: Show that the power series
z 2 z 3
z –  .......
2 3
may be continued analytically to a wider region by means of the series

(1 z) (1 z) 2 (1 z) 3



log 2 –  
2 2 2 2 3 2 3


Solution. The first series converges within the circle C given by |z| = 1 and has the sum
1
function f (z) = log(1 + z). The second series has the sum function
1
  1 z  1 1 z  2 1 1 z  3 





f (z) = log 2 +           .... 
2
   2  2  2  3  2   


  1 z   1 z
= log 2 + log 1     1

  2  2

= log 2 + log   1 z   = log (1 +z)
 2 
1 z

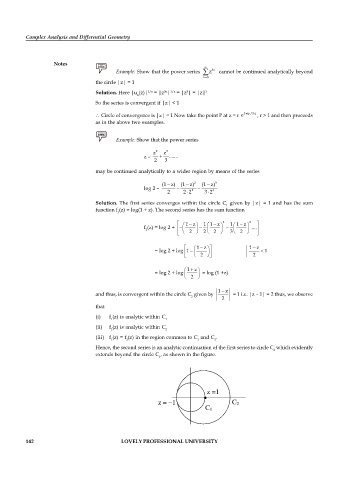
and thus, is convergent within the circle C given by 2 = 1 i.e. |z – 1| = 2 thus, we observe
2
that
(i) f (z) is analytic within C 1
1
(ii) f (z) is analytic within C 2
2
(iii) f (z) = f (z) in the region common to C and C .
1
2
2
1
Hence, the second series is an analytic continuation of the first series to circle C which evidently
2
extends beyond the circle C , as shown in the figure.
1

z =1

z = 1 C 2
C 1

142 LOVELY PROFESSIONAL UNIVERSITY