Page 19 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

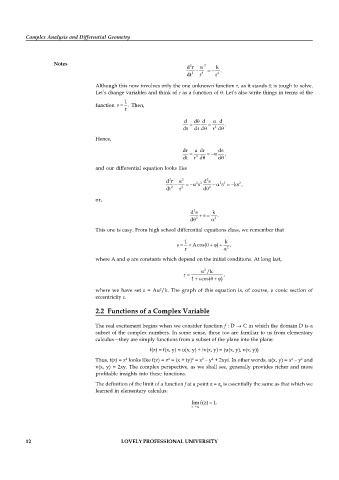
Notes d r  2 k
2
dt 2  r 3   r 2 .

Although this now involves only the one unknown function r, as it stands it is tough to solve.
Let’s change variables and think of r as a function of . Let’s also write things in terms of the
1
function s  . Then,
r

d d d  d .
2
dt  dt d  r d
Hence,
dr a dr ds ,
2
dt  r d   d
and our differential equation looks like

2
2
d r  2 2 2 d s 2 3 ks ,
s
2
dt 2  r 3   d 2   s  
or,
d s k .
2
d 2  s   2
This one is easy. From high school differential equations class, we remember that

1 k
)
s   A cos(    ,
r  2
where A and  are constants which depend on the initial conditions. At long last,

 2 /k
r  ,
1   cos(   )
where we have set  = A /k. The graph of this equation is, of course, a conic section of
2
eccentricity .
2.2 Functions of a Complex Variable

The real excitement begins when we consider function f : D  C in which the domain D is a
subset of the complex numbers. In some sense, these too are familiar to us from elementary
calculus—they are simply functions from a subset of the plane into the plane:

f(z) = f(x, y) = u(x, y) + iv(x, y) = (u(x, y), v(x, y))
Thus, f(z) = z looks like f(z) = z = (x + iy) = x – y + 2xyi. In other words, u(x, y) = x – y and
2
2
2
2
2
2
2
v(x, y) = 2xy. The complex perspective, as we shall see, generally provides richer and more
profitable insights into these functions.
The definition of the limit of a function f at a point z = z is essentially the same as that which we
0
learned in elementary calculus:
lim f(z) L

z 0 z

12 LOVELY PROFESSIONAL UNIVERSITY