Page 17 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

Notes
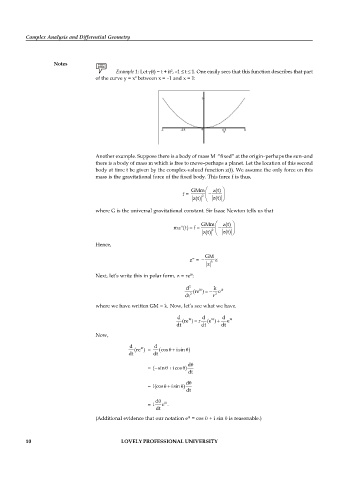
Example 1: Let (t) = t + it , –1  t  1. One easily sees that this function describes that part
2
of the curve y = x between x = –1 and x = 1:
2

Another example. Suppose there is a body of mass M “fixed” at the origin–perhaps the sun–and
there is a body of mass m which is free to move–perhaps a planet. Let the location of this second
body at time t be given by the complex-valued function z(t). We assume the only force on this
mass is the gravitational force of the fixed body. This force f is thus,

GMm  z(t) 
f = 2   
z(t)   z(t)  
where G is the universal gravitational constant. Sir Isaac Newton tells us that

f
2 
mz"(t)   GMm   z(t)  
z(t)   z(t)  
Hence,
GM
z” =  3 z
z
Next, let’s write this in polar form, z = re :
i
d 2 i k e i
dt 2 (re )   r 2
where we have written GM = k. Now, let’s see what we have.

d i r d i d e i
dt (re )  dt (e )  dt
Now,

d (re ) = d isin
i
dt dt cos   

= ( sin   i cos ) d

dt
= i(cos  isin ) d

dt
= i d e .
i
dt
(Additional evidence that our notation e = cos  + i sin  is reasonable.)
i

10 LOVELY PROFESSIONAL UNIVERSITY