Page 12 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Unit 1: Complex Numbers

the so-called triangle inequality. (This inequality is an obvious geometric fact–can you guess Notes
why it is called the triangle inequality?)

1.2 Polar coordinates

Now let’s look at polar coordinates (r, ) of complex numbers. Then we may write z = r(cos  +
i sin ). In complex analysis, we do not allow r to be negative; thus, r is simply the modulus of
z. The number  is called an argument of z, and there are, of course, many different possibilities
for . Thus, a complex numbers has an infinite number of arguments, any two of which differ by
an integral multiple of 2. We usually write  = arg z. The principal argument of z is the unique
argument that lies on the interval (–, ].

Example: For 1 – i, we have

1 – i = 2(cos   7    isin   7   )
 4   4 



= 2(cos      isin     )


 4   4 
= 2(cos   399    isin   399   )
 4   4 

, , and
Each of the numbers 7  399 is an argument of 1 – i, but the principal argument is – .
4 4 4 4
Suppose z = r(cos  + i sin ) and w = s(cos  + i sin ). Then
zw = r(cos  + i sin ) s(cos  + i sin )

= rs[(cos  cos x – sin  sin x) + i(sin  cos  + sin  cos )]
= rs(cos( + ) + i sin ( + )
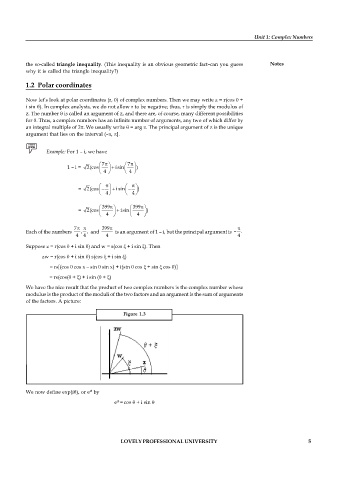
We have the nice result that the product of two complex numbers is the complex number whose
modulus is the product of the moduli of the two factors and an argument is the sum of arguments
of the factors. A picture:

Figure 1.3

We now define exp(i), or e by
i

e = cos  + i sin 
i

LOVELY PROFESSIONAL UNIVERSITY 5