Page 197 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

Notes All calculations in (2) are still in reference to the point P . Though P is a fixed point, it is an
0
0
arbitrary fixed point. Therefore, the equality (2) is valid at any point. Now let’s omit the
intermediate calculations and write (2) as
3
i 
j
E  S e . j ...(3)
i
i 1

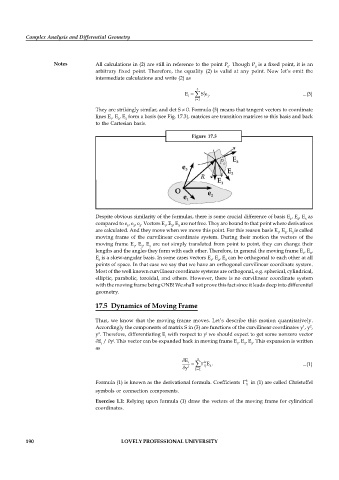
They are strikingly similar, and det S  0. Formula (3) means that tangent vectors to coordinate
lines E , E , E form a basis (see Fig. 17.3), matrices are transition matrices to this basis and back
3
2
1
to the Cartesian basis.
Figure 17.3

Despite obvious similarity of the formulas, there is some crucial difference of basis E , E , E as
2
1
3
compared to e , e , e . Vectors E , E , E are not free. They are bound to that point where derivatives
1
2
2
3
3
1
are calculated. And they move when we move this point. For this reason basis E , E , E is called
3
2
1
moving frame of the curvilinear coordinate system. During their motion the vectors of the
moving frame E , E , E are not simply translated from point to point, they can change their
1
2
3
lengths and the angles they form with each other. Therefore, in general the moving frame E , E ,
1
2
E is a skew-angular basis. In some cases vectors E , E , E can be orthogonal to each other at all
1
2
3
3
points of space. In that case we say that we have an orthogonal curvilinear coordinate system.
Most of the well known curvilinear coordinate systems are orthogonal, e.g. spherical, cylindrical,
elliptic, parabolic, toroidal, and others. However, there is no curvilinear coordinate system
with the moving frame being ONB! We shall not prove this fact since it leads deep into differential
geometry.
17.5 Dynamics of Moving Frame
Thus, we know that the moving frame moves. Let’s describe this motion quantitatively.
Accordingly the components of matrix S in (3) are functions of the curvilinear coordinates y , y ,
1
2
y . Therefore, differentiating E with respect to y we should expect to get some nonzero vector
3
j
i
E / y. This vector can be expanded back in moving frame E , E , E . This expansion is written
j
3
i
2
1
as
3
 E i j   k E . ...(1)

 y k 1 ij k

k
Formula (1) is known as the derivational formula. Coefficients  in (1) are called Christoffel
ij
symbols or connection components.
Exercise 1.1: Relying upon formula (1) draw the vectors of the moving frame for cylindrical
coordinates.
190 LOVELY PROFESSIONAL UNIVERSITY