Page 278 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Unit 22: Bertrand Curves

r Notes
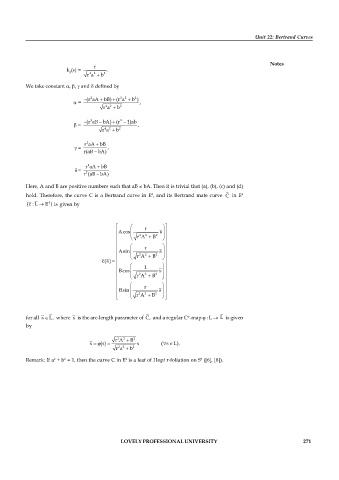
k (s) = .
3
2
4
r a  b 2
We take constant , ,  and  defined by
2
2
2
2

 =  (r aA  bB) (r a  b ) ,
2
4
r a  b 2
2
2


 =  (r aB bA) (r  1)ab ,
2
4
r a  b 2
2
r aA  bB
 = ,
r(aB bA)

4
 = r aA  bB .
2
r (aB bA)

Here, A and B are positive numbers such that aB  bA. Then it is trivial that (a), (b), (c) and (d)
hold. Therefore, the curve C is a Bertrand curve in E , and its Bertrand mate curve C in E 4
4
4

(c : L  E ) is given by
  r  
 Acos s  
2
2
  r A  B 2 
   
  r  
 Asin s  
2
2
  r A  B 2 
c(s)     
  1  
 Bcos s  
2
  r A  B 2 
2
   
  r  
 Bsin  s  
  r A  B 2  
2
2
   
for all s  L, where s is the arc-length parameter of C, and a regular C -map  : L  L is given

by
2
2
r A  B 2
s   (s)  s ( s L).


2
2
r a  b 2
Remark: If a + b = 1, then the curve C in E is a leaf of Hopf r-foliation on S ([6], [8]).
2
3
2
4
LOVELY PROFESSIONAL UNIVERSITY 271