Page 172 - DMTH502_LINEAR_ALGEBRA

Linear Algebra

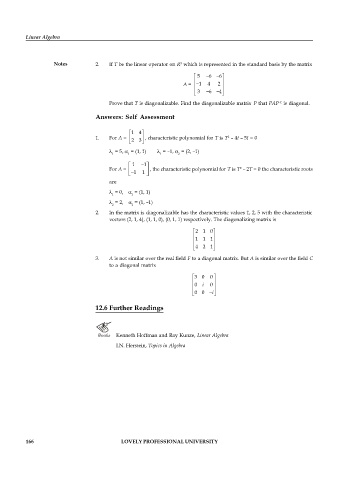
Notes 2. If T be the linear operator on R which is represented in the standard basis by the matrix
3
5 6 6
A = 1 4 2
3 6 4
Prove that T is diagonalizable. Find the diagonalizable matrix P that PAP is diagonal.
–1
Answers: Self Assessment

1 4
2
1. For A = , characteristic polynomial for T is T – 4t – 5I = 0
2 3
= 5, = (1, 1) = –1, = (2, –1)
1 1 1 2
1 1
2
For A = , the characteristic polynomial for T is T – 2T = 0 the characteristic roots
1 1
are
= 0, = (1, 1)
1 1
= 2, = (1, –1)
2 2
2. In the matrix is diagonalizable has the characteristic values 1, 2, 5 with the characteristic
vectors (2, 1, 4), (1, 1, 0), (0, 1, 1) respectively. The diagonalizing matrix is
2 1 0
1 1 1
4 2 1
3. A is not similar over the real field F to a diagonal matrix. But A is similar over the field C
to a diagonal matrix
3 0 0
0 i 0
0 0 i

12.6 Further Readings

Books Kenneth Hoffman and Ray Kunze, Linear Algebra
I.N. Herstein, Topics in Algebra

166 LOVELY PROFESSIONAL UNIVERSITY