Page 188 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Unit 10: Green’s Function Method

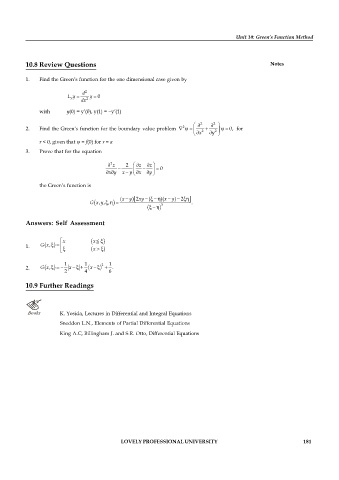
10.8 Review Questions Notes

1. Find the Green’s function for the one dimensional case given by

d 2
L y 2 y 0
x
dx
with y(0) = y (0), y(1) = y (1)

2 2
2. Find the Green’s function for the boundary value problem 2 0, for
x 2 y 2
r < 0, given that = f(0) for r = a
3. Prove that for the equation

2
z 2 z z
0
x y x y x y
the Green’s function is

(x y ) 2xy ( )(x y ) 2
y
G x , , , .
3
Answers: Self Assessment

x x
1. G , x
x
1 1 2 1
2. G , x x x .
2 4 6

10.9 Further Readings

Books K. Yosida, Lectures in Differential and Integral Equations
Sneddon L.N., Elements of Partial Differential Equations

King A.C, Billingham J. and S.R. Otto, Differential Equations

LOVELY PROFESSIONAL UNIVERSITY 181