Page 187 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Differential and Integral Equation

Notes 
Where n is the outward drown normal to the boundary curve C.
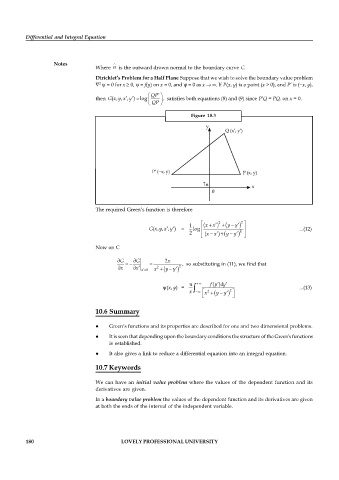
Dirichlet’s Problem for a Half Plane Suppose that we wish to solve the boundary value problem
2 = 0 for x 0, = f(y) on x = 0, and = 0 as x . If P(x, y) is a point (x > 0), and P is ( x, y),
QP
then ( , , , ) logG x y x y , satisfies both equations (8) and (9) since P Q = PQ. on x = 0.
QP

Figure 10.3

The required Green’s function is therefore
2 2
1 x x y y
x
G ( , , , ) = log ...(12)
y
y
x
2 x x y y 2
Now on C
G G 2x , so substituting in (11), we find that
x x x 0 x 2 y y 2

y
f ( )dy
(x, y) = ...(13)
x x 2 y y 2

10.6 Summary

 Green’s functions and its properties are described for one and two dimensional problems.

 It is seen that depending upon the boundary conditions the structure of the Green’s functions
is established.
 It also gives a link to reduce a differential equation into an integral equation.

10.7 Keywords

We can have an initial value problem where the values of the dependent function and its
derivatives are given.
In a boundary value problem the values of the dependent function and its derivatives are given
at both the ends of the interval of the independent variable.

180 LOVELY PROFESSIONAL UNIVERSITY