Page 335 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Differential and Integral Equation

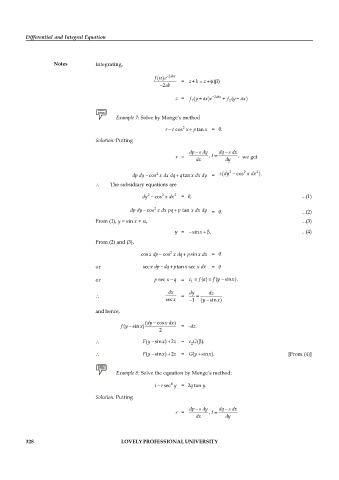
Notes Integrating,

f ( )e 2abx
= z k z ( )
2ab
z = f 1 (y ax e 2abx f 2 (y ax )
)

Example 7: Solve by Monge’s method

2
r t cos x p tanx = 0.
Solution: Putting

dp s dy dq s dx
r = , t , we get
dx dy
2
2
dp dy cos x dx dq q tanx dx dy = ( s dy 2 cos x dx 2 ).
The subsidiary equations are
2
dy 2 cos x dx 2 = 0, ...(1)
2
dp dy cos x dx pq p tan x dx dy = 0. ...(2)
From (1), y = sin x + , ...(3)
y = sin x . ...(4)

From (2) and (3),
2
cosx dp cos x dq p sin x dx = 0
x
or secx dp dq p tan sec x dx = 0
a
x
or p sec x q = c 1 f ( ) ( f y sin ).
dx dy dz
=
secx 1 (y sin )
x
and hence,
(dy cosx dx )
x
( f y sin ) = dz.
2
x
( F y sin ) 2z = c G ( ).
2
x
x
( F y sin ) 2z = G (y sin ). [From (4)]
Example 8: Solve the equation by Monge’s method:

4
t r sec y = 2q tan y.
Solution: Putting
dp s dy dq s dx
r = , t
dx dy

328 LOVELY PROFESSIONAL UNIVERSITY